 Author
Author |
Topic: 0.999... (Read 34430 times) |
|
Icarus
wu::riddles Moderator
Uberpuzzler
    

Boldly going where even angels fear to tread.
Gender: 
Posts: 4863
|
 |
Re: 0.999...
« Reply #125 on: Feb 17th, 2007, 7:26am » |
 Quote Quote  Modify Modify
|
on Feb 17th, 2007, 5:16am, SMQ wrote:What I think is "fun" is that under those definitions, expressions like  - 1, (technically - 1, (technically  - 1) and - 1) and   are meaningful and useful numbers! are meaningful and useful numbers!
Now here's a question I haven't found an answer to: the Wikipedia article states (witout proof, although I believe it to be correct) that where the reals are the "smallest topologically complete ordered field", the surreals are the largest ordered field (if we allow fields to be defined over proper classes/collections as well as sets); does that make the complex surreals (A + B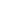 where A and B are surreal numbers and where A and B are surreal numbers and 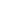 is defined to be is defined to be  -1 as usual) the largest algebraicly closed field? I.e. can all algebraically closed fields be embedded in the complex surreals? -1 as usual) the largest algebraicly closed field? I.e. can all algebraically closed fields be embedded in the complex surreals? |
|
I don't have a definitive answer for this one either, but I doubt it. If we consider an ordered field to be one dimensional, then the surreal complex numbers are 2 dimensional. I suspect that there should be algebraically closed fields that are not 2 dimensional.
In fact, I doubt that the p-adics are embeddable in the surreals.
on Feb 17th, 2007, 5:16am, SMQ wrote:And does 0.999... still equal 1?  |
|
Of course you know, but just so the record is clear for others: In any extension of the real numbers, 0.999... = 1. Otherwise, it couldn't be an extension of the reals.
|
| « Last Edit: Feb 17th, 2007, 8:45am by Icarus » |
 IP Logged IP Logged |
"Pi goes on and on and on ...
And e is just as cursed.
I wonder: Which is larger
When their digits are reversed? " - Anonymous
|
|
|
Icarus
wu::riddles Moderator
Uberpuzzler
    

Boldly going where even angels fear to tread.
Gender: 
Posts: 4863
|
 |
Re: 0.999...
« Reply #126 on: Feb 17th, 2007, 7:43am » |
 Quote Quote  Modify Modify
|
Reading that Wikipedia article, I notice that they define the set S differently than I do. My version has the S for each ordinal including cuts of all older surreals. But they define S differently than I do. My version has the S for each ordinal including cuts of all older surreals. But they define S to simply be the collection of all older surreals, with the cuts added in only for to simply be the collection of all older surreals, with the cuts added in only for  + 1. As a result, all non-dyadic real numbers have birthday + 1. As a result, all non-dyadic real numbers have birthday  + 1 for them, but only + 1 for them, but only  for me. In fact, no surreal has for me. In fact, no surreal has  for a birthday in their scheme, since their S for a birthday in their scheme, since their S is simply a compilation of all that was defined before. is simply a compilation of all that was defined before.
Personally, I prefer my approach (of course  ). Theirs treats limit ordinals differently than others. Their induction has one method to construct S ). Theirs treats limit ordinals differently than others. Their induction has one method to construct S if if 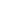 is a limit ordinal, and another method when it is not. My approach has a single method that works no matter type of ordinal we have. Further, every step in my construction introduces new numbers. Limit ordinals do not introduce anything new in their construction. is a limit ordinal, and another method when it is not. My approach has a single method that works no matter type of ordinal we have. Further, every step in my construction introduces new numbers. Limit ordinals do not introduce anything new in their construction.
I'm curious now if their approach is "standard", or did whoever wrote up the wikipedia article misunderstand and introduce an unneeded variation?
|
| « Last Edit: Feb 25th, 2007, 8:13pm by Icarus » |
 IP Logged IP Logged |
"Pi goes on and on and on ...
And e is just as cursed.
I wonder: Which is larger
When their digits are reversed? " - Anonymous
|
|
|
kiochi
Newbie


Posts: 42
|
 |
Re: 0.999...
« Reply #127 on: Mar 21st, 2007, 9:29pm » |
 Quote Quote  Modify Modify
|
Quote:| the surreals are the largest ordered field (if we allow fields to be defined over proper classes/collections as well as sets) |
|
I don't think it really makes sense to talk about the "largest" ordered field if we allow it to be a proper class. For several reasons:
First, any field ought to be a set, as is every mathematical object. Heck, in a math class, even logical symbols like "=" are formally considered to be sets.
The "size" of any mathematical object is the cardinality of it, namely the smallest cardinal that can be put into bijective correspondence with it. Now if we allow a proper class to have a cardinality, then we'll run into something like the Burali-Forti paradox (basically we just contradict the foundation axiom).
Of course we still talk about things that aren't sets, like the collection of all ordinals, "On," but we don't compare these constructs to sets. We can use this construct as a way to do transfinite induction (as Icarus did when defining the surreal numbers). Formally, though these aren't sets, they are basically just logical formulae, e.g. "x is an ordinal," which we can talk about and manipulate only metamathematically.
EDIT: To distinguish a set from a class, note that we are only allowed to construct sets from other sets. e.g. we can take unions of sets, power-sets of sets, as allowed by ZFC. Also, by the replacement schema, we can define a set as a collection with a certain property, but even this requires a set, e.g. given a set X we can form the set {x in X : some property of x holds} (often the "in X" part is left out when the set X is obvious or implicit). Thus we can't write something like: {x: x is an ordinal} because there is no set from which x is to be chosen.
|
| « Last Edit: Mar 21st, 2007, 9:34pm by kiochi » |
 IP Logged IP Logged |
|
|
|
Icarus
wu::riddles Moderator
Uberpuzzler
    

Boldly going where even angels fear to tread.
Gender: 
Posts: 4863
|
 |
Re: 0.999...
« Reply #128 on: Mar 22nd, 2007, 6:03pm » |
 Quote Quote  Modify Modify
|
In ZFC, this is true, as ZFC does not consider the existence of collections other than sets. There are other Set theories that do, however.
Perhaps the simplest example would be NBG, or Von Neumann-Bernays-Godel set theory. This is a conservative extension of ZFC that allows proper classes which cannot themselves be part of any collection. Such classes are actual objects of the theory, not just logical formulas. In NBG, {x : x is an ordinal} makes perfect sense. It is just a proper class, not a set.
Most of the time when mathematicians deal with things like surreals and ordinals and categories that cannot be contained within a set, they will use NBG as their set theory. But in many ways, it has uncomfortable limitations. You cannot build constructions on proper classes, because they cannot be the element of anything. So you cannot speak of "the topology of the surreals" like you can "the topology of the reals", because open subsets of the surreals are all proper classes, and so by NBG cannot be collected together in a larger object, the topology itself.
But NBG is not the only alternative. For example, Russell and Whitehead built an entire pantheon of higher and higher tiers of collections, each of which cannot be the elements of lower tier collections, can be gathered in collections of the same tier in most, but not all, useful fashions (powers, unions, etc), and can always be gathered in higher tier collections. (If that last sentence was confusing to you, then you have a true taste of RW - an extremely influential set of books, but convoluted to an extraordinary level. I'm not sure that anyone other than Russell and Whitehead themselves ever truly understood it.)
A much more modest approach gives us all we need for just about anything people want to do: use a Grothendieck Universe. Start with ZFC (or NBG) and add the following axiom: There is a class U which
1) contains the empty set and all "urelements" (i.e., anything that isn't a collection).
2) for x, y  U, {x, y} U, {x, y}  U. U.
3) for all indexed collections {Sx}x A with A A with A  U, {Sx} U, {Sx} 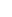 U, U,  x x A Sx A Sx  U. U.
4) for all A  U, A U, A 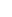 U U
5) for all A  U, P(A) = {x : x U, P(A) = {x : x 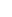 A} A}  U. U.
6) U contains an infinite collection.
The set U is called a Gronthendieck universe (actually 1-5 make it that, the infinite element is necessary for much of mathematics, however). You can restrict the word "Set" to mean an element of U (and not an urelement, if your theory has them), and the word "class" to mean things not in U. At first, this seems like we are restricting our sets to just a portion of what we should have under ZFC. This is not so, though. If we throw out everything not in U (which includes U itself, because ZFC does not allow collections to contain themselves), what we have left is the complete ZFC theory!. Every axiom of ZFC is satisfied by the elements of U alone, without anything more. Further, The elements of U do not add anything to ZFC. If you can prove something about the elements of U that does not involve U itself, then you can prove the same thing in ZFC. Effectively, U is the universe of ZFC, or of NBG. But unlike NBG, you can construct collections that include U as a member. Rather than thinking of U as a restriction of ZFC, the various classes built on U should be considered additions to ZFC.
All of the various definitions made with sets also apply to classes in ZFC + U. The Surreals, ordinals, and cardinals are all actual classes, and you can build upon them to create more classes. The topology of the Surreals is a well-defined object as is its dedekind completion, and the topology of its dedekind completion (an idea impossible to express in NBG). Yet the "class" {A : A  A} does not exist, any more than it does in ZFC. A} does not exist, any more than it does in ZFC.
The downside of ZFC + U is that sethood is not a matter of size, as we are used to thinking. Even the singleton {U} is a proper class, not a set.
One other thing: since we can make these constructions, the temptation is to extend the surreals, ordinals, and other inductive constructions outside of U to classes. This is possible, but it isn't profitable. Since all our classes can be considered ZFC sets (after all, our theory is just ZFC with a special "set" singled out), and since every ZFC set can be considered an element of U, everything we can do by extending Surreals et al to classes, we've already can do within U.
|
| « Last Edit: Mar 25th, 2007, 12:16pm by Icarus » |
 IP Logged IP Logged |
"Pi goes on and on and on ...
And e is just as cursed.
I wonder: Which is larger
When their digits are reversed? " - Anonymous
|
|
|
kiochi
Newbie


Posts: 42
|
 |
Re: 0.999...
« Reply #129 on: Mar 27th, 2007, 11:50am » |
 Quote Quote  Modify Modify
|
Ah yes, I remember reading a set theory book once that used NBG. I don't recall exactly, but it seemed to me that it was just an attempt to expose what people who use ZFC already know: you can form collections, these are called classes. Some classes are called sets. Then you can formally talk about the distinction between sets and classes; still, in ZFC you can talk about classes as well, like the universe V, or the class of all ordinals, and these distinctions are still formal. I guess I just don't see what the advantage of NBG is. It would still be impossible to have the same notion of size for classes and sets, so you could develop a new notion of size for classes, but this you could do equally well within ZFC as NBG.
What's a urelement? If I understand what you are saying, a urelement is "not a collection," it contains nothing. If we're in ZFC (or NBG, I think), then, extensionality says that every "urelement" is 0, but perhaps you aren't using that kind of an axiom?
This U is also new to me, probably becasue I haven't read much about this stuff, but it seems to me to be a perfectly reasonable universe for set theory. You called U a set, though, and for U to be a model of the theory of ZFC, it would have to "think" that it's universe, U, is not a set, right? How does U have to be? It seems to me that U could be very large, e.g. U=V seems reasonable, but then I think U=V_i for some limit ordinal i might also work (in which case, if we took the usual universe V and ZFC, we would say U is a set in the usual model of ZFC).
You say a "downside to ZFC + U is that sethood is not a matter of size." I'm really not sure what you mean by this. What is ZFC + U? Are you just taking a model (U, \in) of ZFC or is U some axiom involving U? I'm not sure what you mean by "sethood" being a "matter of size," but if we have two models of the same theory, then sethood in either of them will be the same (up to isomorphism).
You seem to be astounded that this U can be a model of the entire theory of ZFC. It reminds me of a logic book I read in which they proved using things called skolem functions that there exists a countable model of ZFC. In this book they called it a "paradox," but I didn't think it was. Sure, this structure's universe is only countable, but it still "thinks" it has uncountable elements, which is perfectly fine, it's just that, within the structure, you can't find any bijection from the "countable" elements to the "uncountable" ones (here the quotes indicate that the terms are relativized to the countable model).
|
|
 IP Logged IP Logged |
|
|
|
Icarus
wu::riddles Moderator
Uberpuzzler
    

Boldly going where even angels fear to tread.
Gender: 
Posts: 4863
|
 |
Re: 0.999...
« Reply #130 on: Mar 27th, 2007, 5:56pm » |
 Quote Quote  Modify Modify
|
A urelement is any object in a set theory that is not a set or class or other sort of collection. For instance, in the old "chinese proverb" when you see a horse and a cow, you are actually seeing three things: a horse, a cow, and the set consisting of the horse and the cow, the horse and cow are urelements of the set theory. (Of course, mathematicians count three more things that the proverb misses...)
ZFC does not contain urelements. Nor does NBG or most other set theories. However, you can add axioms and symbols to all of these that would constitute the introduction of urelements. The only change you would need to make is that the axiom of extent only applies to collections (a restriction not needed in the unaltered theory, as everything is a collection there). In fact, the axiom of extent essentially is the definition of the concept of a "collection" - specifying that its identity is completely determined by its elements. Urelements would have their own axioms specifying when you can claim they are equal.
If you choose to add a Grothendieck universe to a set theory that has urelements, then you want all urelements to be a part of your universe.
You cannot set U = V in ZFC, as there is no such object as V in ZFC (as you yourself have said, the universe of ZFC and all proper classes are not actual objects in it, but formal expressions that actually represent logical properties). The whole point of U is that it is an actual object that can be used in other collections and constructions.
V does exist as an actual object in NBG. This is one of the reasons this theory was developed: to have a theory where the things you are pretending are allowable in ZFC when you talk about proper classes actually are allowable. Another advantage of NBG is that is "finitely axiomable". Most set theories, including ZFC, rely upon metamathematical expressions, called axiomatic schema, that are essentially rules that allow you to create an infinite number of actual axioms within the theory itself. NGB, however, can be completely expressed with a finite number of axioms within the theory itself.
However, even though the total universe V exists within NGB, it would be pointless to set U = V. Again, the point of U is to be able to construct with it, as it can be an element of collections. In NBG, proper classes, including V, cannot occur on the left side of the  sign. sign.
For Grothendieck universes, you need more than just a limit ordinal, what you need is a strongly inaccessible cardinal. This a cardinal 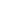 such that for all such that for all 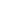 < < 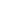 , 2 , 2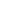 < < 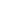 , and , and  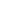 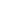 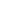 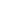 < < 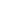 . The existance of a Grothendieck universe is equivalent to the existance of a strongly inaccessible cardinal (neither can be proven or disproven within the confines of ZFC - which is why a Grothendieck universe must be introduced with an axiom). . The existance of a Grothendieck universe is equivalent to the existance of a strongly inaccessible cardinal (neither can be proven or disproven within the confines of ZFC - which is why a Grothendieck universe must be introduced with an axiom).
Yes, when I made the previous post, I accidentally called U a set. I noticed this a couple days ago and changed it to "class", because I want "set" to mean "a collection which is an element of U". In ZFC + U (by which I thought it would be obvious from context means "ZFC with a Grothendieck Universe"), the objects are all "classes", while "set" means a class in U. By this definition, U itself is not a set (in ZFC, no class can be an element of itself).
Concerning size and sethood: In ZFC, any property that is satisfied by only a small number of elements forms a set. In NBG (at least in the version with the axiom of choice), this concept is more explicit: every property determines a class. That class is a set if and only if its size is strictly less than that of V, the set universe. This concept does not hold true in ZFC+U. U cannot be a set. But if A  U, then A U, then A 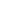 U as well. Therefore {U} is not a set, even though it has only one element. If it were a set, then we would have U U as well. Therefore {U} is not a set, even though it has only one element. If it were a set, then we would have U  U, which is impossible (the axiom of regularity prevents it). U, which is impossible (the axiom of regularity prevents it).
I am not "astounded" that U alone is a model for all of ZFC. (At one time, I would have been, but I have seen this sort of thing far too often to be amazed by it anymore.) My comments about this had a different purpose entirely. Effectively, what U does is chose a particular set in ZFC and limit all sets to it. If I define ordinals or surreals in ZFC, then I define them for all sizes. Nothing stops me until I run into the immovable barrier of logical consistency. However, if I define them in ZFC+U, I choose a particular collection U in ZFC's universe of discourse, and limit my construction to keep it inside U. The natural supposition here is that I don't get the "full surreals" or "full ordinals" this way. I could continue my constructions beyond U and get more ordinals, more surreals. Hence I lose out on these larger objects by limiting myself to U.
What I was trying to show is that this supposition is false. Since U is a model for ZFC, everything I can construct in ZFC+U that does not depend intrinsically on U itself, I can also construct inside of U. My point here is I don't have to worry about missing anything by restricting myself to U. Instead of considering U to be just a portion of ZFC, I can consider U to be all of ZFC, and everything outside of U to be added on to ZFC.
|
| « Last Edit: Mar 27th, 2007, 5:58pm by Icarus » |
 IP Logged IP Logged |
"Pi goes on and on and on ...
And e is just as cursed.
I wonder: Which is larger
When their digits are reversed? " - Anonymous
|
|
|
kiochi
Newbie


Posts: 42
|
 |
Re: 0.999...
« Reply #131 on: Mar 28th, 2007, 10:18am » |
 Quote Quote  Modify Modify
|
Thanks,
That was really interesting to read and it helped me understand to a large extent what you are talking about. It makes sense to me that you would need a strong inaccesible cardinal to have a set like your U, because you don't want to be able to "get out of U" using your sets in U, but why doesn't omega work? For every finite n<omega certainly 2^n<omega, and so on.
And finally one last question, because I am still a little confused about this ZFC+U. The advantage of U seems to be that it's a set in ZFC (that is, maybe, if we assume some large cardinal exists). But then when you're in ZFC+U you say you can sort of restrict sethood to mean "in U" and then do ZFC entirely within U (or am I totally mising the point?). But then U ceases to be a set, at least as far as the this new model is concerned. So what's going on here? Do you have two models of ZFC at the same time, one relativized so that U is the universe? I will stop speculating because I know you will explain it clearly..
Thanks
|
|
 IP Logged IP Logged |
|
|
|
Icarus
wu::riddles Moderator
Uberpuzzler
    

Boldly going where even angels fear to tread.
Gender: 
Posts: 4863
|
 |
Re: 0.999...
« Reply #132 on: Mar 28th, 2007, 7:17pm » |
 Quote Quote  Modify Modify
|
Well, I'll try to explain it clearly, but given that I've failed twice now... 
First of all,  is a strongly inaccessible cardinal, and it does work for the "standard definition" of Grothendieck universe, which does not include my axiom (6). But I want U to be a model for ZFC in its own right. Since ZFC includes the axiom of infinity, U has to contain infinite sets for it to work. For this reason, I added axiom (6): U contains an infinite set. is a strongly inaccessible cardinal, and it does work for the "standard definition" of Grothendieck universe, which does not include my axiom (6). But I want U to be a model for ZFC in its own right. Since ZFC includes the axiom of infinity, U has to contain infinite sets for it to work. For this reason, I added axiom (6): U contains an infinite set.
We add U to ZFC by adding the axioms (1)-(6) to the axioms of ZFC. This is equivalent to assuming the existance of a strongly inaccessible cardinal >  . (i.e., adding an axiom that says such a cardinal exists). . (i.e., adding an axiom that says such a cardinal exists).
What I am doing with U is that I use it break the sets in ZFC into two groups: those sets within U, I still call "sets". Those sets outside U, I no longer refer to as "sets", but only as "classes". Then I argue that this restriction of sethood actually does not cost me anything, as U alone is a model for the full universe of ZFC. The point is to establish a theory of classes that allows me to have classes contained within other classes.
To see the utility, compare the three theories:
- ZFC: all objects are sets. Some relations determine sets, others do not. Relations that do not form sets can only be "collectivized" formally: we pretend that they can be, and refer to the collections as "classes". But these classes do not really exist, and so we cannot reference them in any way within our theory.
- NBG: All objects are classes. Classes that are smaller than the Universe are sets. Proper classes (all classes the same size as the universe) cannot be used on the left side of the
 sign, so they cannot ever be the members of other classes. All relations may be collectivized, but the resulting class will contain only the sets (no classes) that satisfy the relation. For instance, the class V = {x : x = x}, called the Set Universe, does not contain itself, even though V = V. sign, so they cannot ever be the members of other classes. All relations may be collectivized, but the resulting class will contain only the sets (no classes) that satisfy the relation. For instance, the class V = {x : x = x}, called the Set Universe, does not contain itself, even though V = V.
- ZFC+U: All objects are classes. Those that are in U are sets. Proper classes can be contained in other proper classes. Not all relations determine classes, but all relations restricted to sets do determine classes (for example, {x : x is a set and x
 x} = U, which avoids Russell's paradox since U is not a set). Since classes may be elements of other classes, normal mathematical constructions may be used without any hemming or hawing. x} = U, which avoids Russell's paradox since U is not a set). Since classes may be elements of other classes, normal mathematical constructions may be used without any hemming or hawing.
For example, consider the order topology of the Surreals. Every interval in the surreals contains a subclass that is order-isomorphic to the ordinals. Therefore every non-empty open class of surreals is not a set.
- ZFC: The surreals themselves cannot be collected. They are instead determined by a relation that we can translate to be "is a surreal number". The same is true of open classes within the surreals. The concept that the union of open classes is itself an open class is not even expressible in ZFC. Neither is the concept of the "Topology of the surreals" (i.e., all the open classes of the surreals).
- NBG: The surreals exist as an object, as do all the open classes. The concept that the union of open classes is itself an open class is expressible for any fixed finite number of classes, but is not expressible for infinite numbers of classes, or even as a single rule that applies for all finite classes. The topology of the surreals may only be referred to formally. It does not exist as an object.
- ZFC+U: The surreals and all open classes exist, as does the topology itself, and the class of all topologies on the surreals, and whatever other class I'd like to deal with (to within the same limitations that always apply in set theories). That the union of any class of open classes is also an open class is no more of a problem to express than the same concept for sets. Because ZFC+U is built from ZFC, anything I can do with a set in ZFC, I can do with a class in ZFC+U.
I hope that gives you an idea of why one would want to use a Grothendieck universe. It makes dealing with collections too large to be sets no harder than dealing with sets themselves. If I use a Grothendieck universe, I have no need to worry about the difference between "fields" and "big fields" - the latter being a field that is a class instead of a set. If I don't use ZFC+U, or some other set theory that allows building on classes, then I have to define the concept of a "big field" somewhat differently than that of a field (the basic concepts are the same - but the formalism has to be adapted to not refer to sets).
Of all the set theories that allow you to build on classes, ZFC+U strikes me as by far the simplest. Yet it is more than sufficient for anything I'm likely to need.
|
| « Last Edit: Mar 28th, 2007, 8:03pm by Icarus » |
 IP Logged IP Logged |
"Pi goes on and on and on ...
And e is just as cursed.
I wonder: Which is larger
When their digits are reversed? " - Anonymous
|
|
|
Product
Newbie


x * y
Posts: 10
|
 |
Re: 0.999...
« Reply #133 on: Apr 26th, 2007, 12:37pm » |
 Quote Quote  Modify Modify
|
I will start off this post admitting that much of the math and mathematical theory in this thread is above me. I post not to debate or offer my own solution, but to have the flaws in my thinking pointed out to me. I've always stayed out of the whole debate because I'm not a number theory wizard. I do teach elementary school though, and while teaching a little about probability the other day, a thought occurred to me.
If you flip a fair, two headed coin where the odds are exactly 1:1 that it will land heads, and you plan to flip it an infinite number of times, the probability that it will land heads at least once is .999~ but it is not 1 because the coin never has to come up heads. Theoretically it could land tails every time out into infinity, regardless of the realistically non existant chance that it would even happen out to 100.
So that's the thought I had. I'm sure there are flaws and I just want to see where my reasoning is wrong. Is it that probability can't accurately be measured in decimals? Am I comparing wrong types of numbers? My best guess is that the .999~ probability comes from 1-1/(infinity), and you can't divide by infinity, so the whole idea of .999~ being a probability is silly anyway. Thanks for any discussion or answers.
|
|
 IP Logged IP Logged |
|
|
|
Sir Col
Uberpuzzler
    

impudens simia et macrologus profundus fabulae

Gender: 
Posts: 1825
|
 |
Re: 0.999...
« Reply #134 on: Apr 26th, 2007, 3:33pm » |
 Quote Quote  Modify Modify
|
I'll have a go at addressing your query. It seems that the problem comes down to the difference between infinite and finite.
To make a better comparison with 0.999..., consider the following experiment.
A bag contains nine red discs and one blue disc. A disc is taken at random and its colour is noted. The disc is returned and the experiment is repeated N times.
Therefore P(R=0) = 1/10N.
Hence P(R>0) = 1-1/10N.
N=1: P(R>0) = 0.9
N=2: P(R>0) = 0.99
N=3: P(R>0) = 0.999
et cetera.
Now for any finite number of times the experiment is repeated, N, the resulting probability will be zero point followed by a finite string of N nines.
If the experiment is repeated forever, then we move from a finite set of possible outcomes: 0 reds, 1 red, 2 reds, 3 reds, ..., N reds, to an infinite (continuous) set; there is nothing in-between. So the probability of getting no reds is zero by definition. In fact, P(R=0) = P(R=1) = P(R=2) = ... = P(R=N) = 0; for any continuous data, P(R=r) = 0. Hence P(R>0) = P(R>=0) = 1.
The problem is that we try to extend the finite cases as N increases to the realm of the infinite as if the infinite case is the next one after the "last" finite case. The reality in the context of probability is that we are dealing with an either/or situation. We have a finite number of cases (discrete), in which case there is a finite string of nines; or we have the infinite case (continuous), in which case we have unity (or zero).
|
|
 IP Logged IP Logged |
mathschallenge.net / projecteuler.net
|
|
|
Icarus
wu::riddles Moderator
Uberpuzzler
    

Boldly going where even angels fear to tread.
Gender: 
Posts: 4863
|
 |
Re: 0.999...
« Reply #135 on: Apr 26th, 2007, 4:18pm » |
 Quote Quote  Modify Modify
|
An insightful question, but what it points out is the limitations of the concept of probability, and not of the fact that 0.999~ = 1.
What you have to understand is that when there are an infinite number of possible outcomes, saying that a particular outcome has probability 0 does not mean that the outcome cannot occur. And saying that the outcome ("event", in the language of probability theory) has probability 1 does not guarantee that the event will occur.
For example: consider the case of picking a real number between 0 and 1 at random (i.e., with uniform probability). For any two numbers a and b with 0 <= a < b <=1, the probability that the number x picked lies between a and b (a <= x <= b), is b - a. So if I choose any particular number N and ask what is the probability that N will be picked, the answer must be exactly 0: If P(N) > 0, then I could note that N lies between (N-P(N)/4) and (N+P(N)/4), but the probability that the number picked is between (N-P(N)/4) and (N+P(N)/4) is only P(N)/2, which cannot be. Therefore we must have P(N) = 0.
This holds for every number N between 0 and 1. Each individually has exactly 0 probability of being picked. So every time a pick is made, it was made despite the probability being 0.
Note that the argument does not allow for P(N) to be some number "just slightly above zero" in any fashion at all. No matter how close P(N) is to 0, if it is not actually 0, it leads to the conclusion that N is more likely to be picked itself than any number is out of a set to which N belongs.
[edit] This is what happens when you take a break in the middle of composing a post! Someone slips in ahead of you![/edit]
|
| « Last Edit: Apr 26th, 2007, 4:19pm by Icarus » |
 IP Logged IP Logged |
"Pi goes on and on and on ...
And e is just as cursed.
I wonder: Which is larger
When their digits are reversed? " - Anonymous
|
|
|
Grimbal
wu::riddles Moderator
Uberpuzzler
    

Gender: 
Posts: 7526
|
 |
Re: 0.999...
« Reply #136 on: Apr 27th, 2007, 1:30am » |
 Quote Quote  Modify Modify
|
This reminds me of the following paradox.
Ask what proportion of all integers can be written in decimal without the digit 3?
For up to n-digit numbers, the proportion is is (9/10)n. At the limit for n->inf, it is 0.
The conclusion is that 0% of all integers can be written without the digit 3.
Does it mean there are none? Of course not, I can think of plenty of them.
Does it mean, then, that the limit isn't really 0? No, limn->inf (0.9)n is exactly 0. The problem comes from the fact that you cannot compare the size of infinite sets in this way.
|
|
 IP Logged IP Logged |
|
|
|
TruthlessHero
Newbie




Gender: 
Posts: 40
|
 |
Re: 0.999...
« Reply #137 on: Jun 8th, 2007, 8:15am » |
 Quote Quote  Modify Modify
|
Is there any way you could edit the original posts so they didn't have the smiley stuff? Not sure if since it's so old that it wouldn't work?
|
|
 IP Logged IP Logged |
|
|
|
towr
wu::riddles Moderator
Uberpuzzler
    

Some people are average, some are just mean.
Gender: 
Posts: 13730
|
 |
Re: 0.999...
« Reply #138 on: Jun 8th, 2007, 1:45pm » |
 Quote Quote  Modify Modify
|
on Jun 8th, 2007, 8:15am, TruthlessHero wrote:| Is there any way you could edit the original posts so they didn't have the smiley stuff? Not sure if since it's so old that it wouldn't work? |
|
The postsize limit has changed in the meanwhile, so it would not be possible to save the changes.
|
|
 IP Logged IP Logged |
Wikipedia, Google, Mathworld, Integer sequence DB
|
|
|
javacodeman
Newbie


Gender: 
Posts: 7
|
 |
Re: 0.999...
« Reply #139 on: Jul 6th, 2007, 11:08am » |
 Quote Quote  Modify Modify
|
Mentor a guest wrote in the original Thread (#2)
Quote:mentor
Guest
Re: 0.999.
« Reply #1 on: Jul 26th, 2002, 11:40am »
------------------------------------------------------------------------ --------
An Addendum: it's a short step (left as an excercise to the reader) to show that 1/(ifinity) is 0. |
|
I had to write that this is incorrect as infinity is not a number to be used that way. It is correct to say,
lim (x -> infinity) 1/x = 0.
while,
lim (x -> +0) 1/x = infinity
lim (x -> -0) 1/x = -infinity
java
|
|
 IP Logged IP Logged |
|
|
|
srn437
Newbie


the dark lord rises again....
Posts: 1
|
 |
Re: 0.999...
« Reply #140 on: Aug 29th, 2007, 12:38pm » |
 Quote Quote  Modify Modify
|
Four words: geometric series.
|
|
 IP Logged IP Logged |
|
|
|
CHIMELA
Newbie


Posts: 10
|
 |
Re: 0.999...
« Reply #141 on: Nov 22nd, 2007, 5:34pm » |
 Quote Quote  Modify Modify
|
I remember our teacher told our class about how you take an infinite amount of half-steps whenever you walk to any given place. For example, if you walk towards a stool, first you must walk half the distance to that stool. But you must walk half the distance of that half. And the half of that half. Thus, you walk an infinite amount of half-steps.
I've been wondering something, though. (And keep in mind I'm still in secondary education.) If 1=0.999... and you multiply both sides by 2, would you get 2=1.999...8, or is that not allowed because you can't have an infinite amount of decimals and then have a finite number at the end, so you'd have to simplify 0.999... to 1 first?
|
|
 IP Logged IP Logged |
|
|
|
ThudnBlunder
wu::riddles Moderator
Uberpuzzler
    

The dewdrop slides into the shining Sea
Gender: 
Posts: 4489
|
 |
Re: 0.999...
« Reply #142 on: Nov 22nd, 2007, 5:49pm » |
 Quote Quote  Modify Modify
|
on Nov 22nd, 2007, 5:34pm, CHIMELA wrote:| I remember our teacher told our class about how you take an infinite amount of half-steps whenever you walk to any given place. For example, if you walk towards a stool, first you must walk half the distance to that stool. But you must walk half the distance of that half. And the half of that half. Thus, you walk an infinite amount of half-steps. |
|
Yes, see this link.
on Nov 22nd, 2007, 5:34pm, CHIMELA wrote:| ...or is that not allowed because you can't have an infinite amount of decimals and then have a finite number at the end... |
|
That is correct.
|
|
 IP Logged IP Logged |
THE MEEK SHALL INHERIT THE EARTH.....................................................................er, if that's all right with the rest of you.
|
|
|
steamypi
Newbie


Gender: 
Posts: 2
|
 |
Re: 0.999...
« Reply #144 on: Aug 5th, 2012, 1:59pm » |
 Quote Quote  Modify Modify
|
What I would say is:
First think of this separately,
1/3 = .333333(infinite # of 3's)
OK,
1/3 + 1/3 + 1/3 =3/3
3/3 = 1
RIGHT? YES
SO THEN,
1/3 = .333333(infinite # of 3's)
1/3 = .333333(infinite # of 3's)
+1/3 = .333333(infinite # of 3's)
______
3/3 = 1
So when you say .3333(infinite # of 3's) +.3333(infinite # of 3's) +.3333(infinite # of 3's)=.999999(infinite # of 9's), that's not true because it really equals 1 instead of 999999(infinite # of 9's).
|
| « Last Edit: Aug 5th, 2012, 1:59pm by steamypi » |
 IP Logged IP Logged |
|
|
|
steamypi
Newbie


Gender: 
Posts: 2
|
 |
Re: 0.999...
« Reply #145 on: Aug 5th, 2012, 2:45pm » |
 Quote Quote  Modify Modify
|
And what you must remember is that .333333(infinite # of 3's) is actually 1/3, although many people treat .333333(infinite # of 3's) as something you can add like .333+.333+.333=.999.
Using these numbers that continue infinitely is like rounding a number.
ex.
Say you add like this:
Here's the equation,
.7845
+.4839
_______
But these numbers are too long for you so you round. .7845~.785 and .4839~.484
Now we have:
.785
+.484
________
Add it up and you get 1.269
But when you round like that, the solution is not the actual solution, nor are the numbers that were added together. The actual solution, using the original numbers, is 1.2684
So when you say that .999(infinite # of 9's) is equal to 1, it is like saying, using the example, that 1.269 equals 1.2684.
|
|
 IP Logged IP Logged |
|
|
|
towr
wu::riddles Moderator
Uberpuzzler
    

Some people are average, some are just mean.
Gender: 
Posts: 13730
|
 |
Re: 0.999...
« Reply #146 on: Aug 5th, 2012, 10:09pm » |
 Quote Quote  Modify Modify
|
on Aug 5th, 2012, 2:45pm, steamypi wrote:
So when you say that .999(infinite # of 9's) is equal to 1, it is like saying, using the example, that 1.269 equals 1.2684. |
|
No it's not, because 1.269 is only an approximation of 1.2684 whereas 0.999... is not an approximation at all, it is precisely 1. There is no difference at all between 1 and 0.999..., but the difference between 1.269 and 1.2686 is 0.0004.
Also 0.999... = sum i=1..inf 9*10-i = 3 * sum i=1..inf 3*10-i = 3 * 0.333... So it 3 * 1/3 is 0.999... and it is equal to 1.
0.999... = sum i=1..inf 9*10-i = lim i->inf 1 -1/10i = 1, or actually read the thread to find a hundred other ways to conclude the same simple truth.
|
|
 IP Logged IP Logged |
Wikipedia, Google, Mathworld, Integer sequence DB
|
|
|
marsh8472
Newbie


Posts: 27
|
 |
Re: 0.999...
« Reply #147 on: Sep 15th, 2012, 1:05am » |
 Quote Quote  Modify Modify
|
There's a good chance this has already been brought up but there's an axiom called the Archimedean property for real numbers which states there's no such thing as an infinitesimal number or infinitely large number.
Arguing that 0.99999... < 1 violates that property. I'm sure it's possible to use a different system of math that assumes the Archimedean property does not apply to real numbers though. But this is just an argument in circles otherwise.
|
|
 IP Logged IP Logged |
|
|
|
Edward_E
Newbie


Posts: 10
|
 |
Re: 0.999...
« Reply #148 on: Nov 14th, 2012, 3:59pm » |
 Quote Quote  Modify Modify
|
I like thinking of this problem a little differently.
1 / 1111 = 0.000900090009...
1 / 111 = 0.009009009...
1 / 11 = 0.09090909...
Based on this pattern one would assume that.
1/1 = 0.999...
So I believe that.
1 = 0.999...
This inverse of numbers can be found also in
1/2222 = 0.0004500450045...
1/222 = 0.0045045045...
1/22 = 0.045454545...
1/2 = 0.4999... = 0.5
|
|
 IP Logged IP Logged |
|
|
|
Pavel
Newbie



Posts: 1
|
 |
Re: 0.999...
« Reply #149 on: Feb 25th, 2013, 10:41pm » |
 Quote Quote  Modify Modify
|
amuse we have the secession of real numbers,
S = {a(i) such that a(1)=0.9 and a(n+1)=a(n)+9*10^-(n+1):n=1,2,3,...}
So for every a(i) of S there must exist some number number [Epsilon](a(n))>0 such that for any n=1,2,3,...
1-[Epsilon]=a(n)
thus
1=a(n)+[Epsilon]
since [Epsilon] is a positive real number
1>a(n)
and
1>a(i) for all a(i) belonging to S
therefore
.999...<1
.....
thoughts:
....
.999..... is a concept not a number and its unfair to compare it to the number 1 and not the concept of 1 (thus most proofs above). the discussion i believe is not about limits or convergence but one of a number that looks like this: 0.9999999999999999999999999999
but there is always one bigger but with the trait that it MUST start 0.9..... , and so never quiet 1.
the series S contains infinitely many elements and it does converge to 1 but there isn't a single number in there like 1.
|
| « Last Edit: Feb 25th, 2013, 10:50pm by Pavel » |
 IP Logged IP Logged |
|
|
|
|
 WRITE MATH!
WRITE MATH!
 Home
Home  Help
Help  Search
Search  Members
Members  Login
Login  Register
Register WRITE MATH!
WRITE MATH!
 Home
Home  Help
Help  Search
Search  Members
Members  Login
Login  Register
Register