 Author
Author |
Topic: A Beetle on a Hula Hoop (Read 16349 times) |
|
rloginunix
Uberpuzzler
    

Posts: 1029
|
 |
A Beetle on a Hula Hoop
« on: Dec 4th, 2016, 5:59pm » |
 Quote Quote  Modify Modify
|
A Beetle on a Hula Hoop
A thin hula hoop of radius R and mass M rests on a frictionless horizontal plane.
On the hula hoop there sits a beetle of mass m.
What trajectory will the beetle and the center of the hula hoop trace once the beetle starts crawling along the hula hoop?
(the hoop may be considered to be a massive circumference of uniform density)
|
|
 IP Logged IP Logged |
|
|
|
dudiobugtron
Uberpuzzler
    

Posts: 735
|
 |
Re: A Beetle on a Hula Hoop
« Reply #1 on: Dec 5th, 2016, 12:00am » |
 Quote Quote  Modify Modify
|
Thoughts:
For any particular contraption that gains momentum by gaining purchase on the floor, the centre of gravity will not move relative to the floor if it is frictionless. So, however the beetle moves, the Hula Hoop will move to keep the centre of gravity of the combined system in the same location.
|
|
 IP Logged IP Logged |
|
|
|
alien2
Uberpuzzler
    

Gender: 
Posts: 6986
|
 |
Re: A Beetle on a Hula Hoop
« Reply #2 on: Dec 5th, 2016, 11:03am » |
 Quote Quote  Modify Modify
|
Only the bug is interested in trajectory because Hula Hoop is not alive. Bugs have feelings too. Do they? Do you really think that bugs like when insectologists invade their privacy and then riddlers make fools out of them? Repent, and thou shalt be saved.
|
|
 IP Logged IP Logged |
|
|
|
towr
wu::riddles Moderator
Uberpuzzler
    

Some people are average, some are just mean.
Gender: 
Posts: 13730
|
 |
Re: A Beetle on a Hula Hoop
« Reply #3 on: Dec 5th, 2016, 11:08am » |
 Quote Quote  Modify Modify
|
on Dec 5th, 2016, 12:00am, dudiobugtron wrote:Thoughts:
For any particular contraption that gains momentum by gaining purchase on the floor, the centre of gravity will not move relative to the floor if it is frictionless. So, however the beetle moves, the Hula Hoop will move to keep the centre of gravity of the combined system in the same location.
|
|
That sounds plausible, so the beetle and center of the hula hoop would orbit the common center of gravity.
... barring air resistance .. and the jerky nature of the beetle's crawling .. and non-perfect rigidity of the hula hoop.
|
|
 IP Logged IP Logged |
Wikipedia, Google, Mathworld, Integer sequence DB
|
|
|
rloginunix
Uberpuzzler
    

Posts: 1029
|
 |
Re: A Beetle on a Hula Hoop
« Reply #4 on: Dec 6th, 2016, 8:05am » |
 Quote Quote  Modify Modify
|
dudiobugtron and towr are correct. The law of conservation of linear momentum applies (why).
The orbits' sizes can be computed.
(the little cutesy is a perfect candidate for a Cinderella animation, though I do not have the time for that at the moment)
|
|
 IP Logged IP Logged |
|
|
|
dudiobugtron
Uberpuzzler
    

Posts: 735
|
 |
Re: A Beetle on a Hula Hoop
« Reply #5 on: Dec 6th, 2016, 9:56am » |
 Quote Quote  Modify Modify
|
on Dec 6th, 2016, 8:05am, rloginunix wrote:| The law of conservation of linear momentum applies (why). |
|
Quote:| The orbits' sizes can be computed. |
|
Would it also depend on the beetle's velocity (or corresponding centripetal acceleration)? I know that in a regular orbiting system, the velocity is related to orbit radius - but for the beetle it can actually choose different velocities (as long as it doesn't reach 'escape' velocity) without affecting its orbit radius. I think that might give a different angular momentum.
|
| « Last Edit: Dec 6th, 2016, 10:04am by dudiobugtron » |
 IP Logged IP Logged |
|
|
|
rloginunix
Uberpuzzler
    

Posts: 1029
|
 |
Re: A Beetle on a Hula Hoop
« Reply #6 on: Dec 6th, 2016, 2:47pm » |
 Quote Quote  Modify Modify
|
The essence of the LoCoLM - while individually the momentums of parts may be arbitrarily complicated (functions of time), the vector sum of all the parts' momentums, as overall system's total, must remain constant (over time), zero in this case.
So, since towr pretty much spelled out the answer, all that remains to do is to compute the distances from the system's center of mass to the beetle and to the hoop's center respectively.
|
|
 IP Logged IP Logged |
|
|
|
dudiobugtron
Uberpuzzler
    

Posts: 735
|
 |
Re: A Beetle on a Hula Hoop
« Reply #7 on: Dec 6th, 2016, 3:48pm » |
 Quote Quote  Modify Modify
|
Oops! I did not intend to just post that first quote with no reply to it. I initially had an answer to your question written in my post; I must have accidentally deleted or removed it somehow.
My answer was that there are no external forces acting on the system.
Thanks for your reply, though; I do now see that the orbit radii must be determined exactly by the distances of the components from system's centre of gravity.
|
| « Last Edit: Dec 6th, 2016, 3:53pm by dudiobugtron » |
 IP Logged IP Logged |
|
|
|
rloginunix
Uberpuzzler
    

Posts: 1029
|
 |
Re: A Beetle on a Hula Hoop
« Reply #8 on: Dec 7th, 2016, 11:44am » |
 Quote Quote  Modify Modify
|
Yes.
Yes.
Equivalence (extra credit): if we were to craft a "linear" equivalent of this problem, what might it be?
|
|
 IP Logged IP Logged |
|
|
|
rmsgrey
Uberpuzzler
    

    
Gender: 
Posts: 2872
|
 |
Re: A Beetle on a Hula Hoop
« Reply #9 on: Dec 7th, 2016, 2:24pm » |
 Quote Quote  Modify Modify
|
on Dec 7th, 2016, 11:44am, rloginunix wrote:Yes.
Yes.
Equivalence (extra credit): if we were to craft a "linear" equivalent of this problem, what might it be? |
|
Plane on a treadmill? 
|
|
 IP Logged IP Logged |
|
|
|
rloginunix
Uberpuzzler
    

Posts: 1029
|
 |
Re: A Beetle on a Hula Hoop
« Reply #10 on: Dec 8th, 2016, 6:37am » |
 Quote Quote  Modify Modify
|
 , yep - with one thoroughly confused bug on it. , yep - with one thoroughly confused bug on it.
2) the original setup except that a beetle sits on an edge of a massive uniform line segment (twig) of length L. How far will the segment move if the beetle crawls to its opposite edge?
3) same as 2) plus there's a second beetle of mass m2 on the opposite edge. The beetle m1 crawls to the dead center of the twig. How far along the twig must the beetle m2 crawl so that the twig moves back to its original position?
|
|
 IP Logged IP Logged |
|
|
|
Grimbal
wu::riddles Moderator
Uberpuzzler
    

Gender: 
Posts: 7527
|
 |
Re: A Beetle on a Hula Hoop
« Reply #11 on: Dec 9th, 2016, 1:58am » |
 Quote Quote  Modify Modify
|
The center of mass CM is the weighted average of the positions of the hoop center and the bug navel.
That CM doesn't move. Provided hoop and bug where initially at rest.
The distance between hoop center and bug is constant. So the distance between the CM and the bug is constant at R·M/(M+m).
So, the bug moves along a circle with radius R·M/(M+m).
Similarily, the hoop center moves around a circle of radius R·m/(M+m) around the CM.
The only tricky thing is to find out the actual speed of the bug, given the speed of the bug on the hoop. How many times does a bug have to walk a hoop, until it did a full turn?
PS: as towr summarized quite well...
|
| « Last Edit: Dec 9th, 2016, 2:01am by Grimbal » |
 IP Logged IP Logged |
|
|
|
rloginunix
Uberpuzzler
    

Posts: 1029
|
 |
Re: A Beetle on a Hula Hoop
« Reply #12 on: Dec 9th, 2016, 8:23pm » |
 Quote Quote  Modify Modify
|
Nice work.
Consider 2) as a linear equivalent of Grimbal's problem.
wrt = with respect to
u = velocity of the beetle wrt the twig
v = velocity of the twig wrt the lab frame (plane)
w = velocity of the beetle wrt the frame
By the law/principle of summation of the velocities, in vector form:
(1) w = u + v
In projections on the chosen axis (1) becomes:
(2) w = u - v
In the horizontal plane the total momentum is conserved. It was 0 initially and so is at any instance of time after the parts begin to move:
(3) 0 = mw - Mv
or
(4) mw = Mv
and
(5) w = v M/m
From (2) and (3) we obtain:
mu - mv - Mv = 0
mu - (M + m)v = 0
(6) v/u = m/(M + m)
The travel time 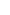 is obviously the time it took the beetle to crawl L with u: is obviously the time it took the beetle to crawl L with u:
(7) 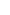 = L/u = L/u
Then, the distance d(t, f) covered by the twig wrt the frame is:
(8) d(t, f) = 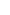 v = Lv/u = Lm/(M + m) (from (6)) v = Lv/u = Lm/(M + m) (from (6))
The distance d(b, f) covered by the beetle wrt the frame is (with (5)):
(9) d(b, f) = 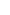 w = Lw/u = L(M/m) (v/u) = L(M/m)(m/(M + m)) = LM/(M + m) w = Lw/u = L(M/m) (v/u) = L(M/m)(m/(M + m)) = LM/(M + m)
We need to take some velocity as known. Grimbal suggests it should be u. So:
(10) v = u m/(M + m)
(11) w = (M/m)v = u M/(M + m)
Goes without saying that (8)/(9) and (10)/(11) are given in the scalar form. In vector form one from each pair must be taken with the opposite sign.
|
|
 IP Logged IP Logged |
|
|
|
rloginunix
Uberpuzzler
    

Posts: 1029
|
 |
Re: A Beetle on a Hula Hoop
« Reply #13 on: Dec 10th, 2016, 8:59am » |
 Quote Quote  Modify Modify
|
Grimbal's problem, where we will use the law of conservation of angular momentum, actually highlights an interesting generic difference between the "linear forward" and rotational motions.
|
|
 IP Logged IP Logged |
|
|
|
rloginunix
Uberpuzzler
    

Posts: 1029
|
 |
Re: A Beetle on a Hula Hoop
« Reply #15 on: Dec 14th, 2016, 9:16am » |
 Quote Quote  Modify Modify
|
Good enough, thanks.
Grimbal's problem: all the velocities are constant and << c, no slippage (as a vector, where does the force of friction is pointing and why?). CCoM = Common Center of Mass, as opposed to hoop's CoM O; LoCoAM = Law of Conservation of Angular Momentum.
Let  1 be the beetle's (absolute) angular velocity wrt the plane (lab frame placed in CCoM). Since there are only two point masses and the hoop is an absolutely rigid circumference, the three points in question - beetle, CCoM and O - will remain collinear at all times. Therefore, the angular velocity of the hoop's CoM O wrt the CCoM is equal to that of the beetle's, 1 be the beetle's (absolute) angular velocity wrt the plane (lab frame placed in CCoM). Since there are only two point masses and the hoop is an absolutely rigid circumference, the three points in question - beetle, CCoM and O - will remain collinear at all times. Therefore, the angular velocity of the hoop's CoM O wrt the CCoM is equal to that of the beetle's,  1. To these two we must also add the angular momentum of the hoop rotating about its CoM O with an angular velocity 1. To these two we must also add the angular momentum of the hoop rotating about its CoM O with an angular velocity  2 so that the LoCoAM's equation becomes: 2 so that the LoCoAM's equation becomes:
(1) 0 = mR2M2 1/(M+m)2 + MR2m2 1/(M+m)2 + MR2m2 1/(M+m)2 + MR2 1/(M+m)2 + MR2 2 2
At any time during the motion the beetle belongs to two circumferences at once: that of the (spinning) hoop and that of its trajectory about the CCoM. Hence:
(2)  = =  1 - 1 -  2 2
where  is the beetle's velocity wrt the hoop - known as per Grimbal's suggestion. is the beetle's velocity wrt the hoop - known as per Grimbal's suggestion.
Solving (1) and (2) for  1 and 1 and  2, in terms of 2, in terms of  , we obtain: , we obtain:
(3)  1 = 1 =  (M+m)/(M+2m) (M+m)/(M+2m)
(4)  2 = - 2 = -  m/(M+2m) m/(M+2m)
Back of the envelope analysis: time has the tendency to prove our most basic assumptions wrong - I am ready to stand corrected in the future, but as of this writing objects' masses in this universe tend to be non zero real numbers. Hence, the  's coefficient is bound from above by 1 in (3) and by 1/2 in (4). 's coefficient is bound from above by 1 in (3) and by 1/2 in (4).
If the beetle is feather light and the hoop is very heavy then m can be almost thrown out of (3) and we see that the beetle's velocity wrt the ground will be almost the same as his/her velocity wrt the hoop, in the limit - it is almost as if the beetle is running along a stationary platform.
Conversely, if the beetle is as fat as a cat and the hoop is feather light then m dominates the ratio and the beetle gets a good cardiovascular workout.
In general: barring the case of an intelligent menacing beetle who, laughing at and mocking us, just flaps his/her legs about faking the motion (and perhaps using its wings to stay up in the air), is it possible for a beetle to run along the hoop but remain stationary wrt a chosen point on the ground?
Timings: in general, by definition, for the angle of turn  we have: d we have: d (t)/dt = (t)/dt =  (t). Multiply both sides by dt and integrate over angle and time. Since the velocities are constant, we have a general formula: (t). Multiply both sides by dt and integrate over angle and time. Since the velocities are constant, we have a general formula:  = =  t + Const, where Const may be chosen to be zero. t + Const, where Const may be chosen to be zero.
The time it takes the beetle to run once around the hoop is:
(5) 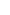 = 2 = 2 / /
Consequently, the angle covered by the beetle as it moves around CCoM (in that time) is:
(6)  1 1 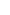
To make a full turn around CCoM the above angle must be 2 and and 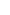 must be multiplied by x - the number in Grimbal's question. must be multiplied by x - the number in Grimbal's question.
Lastly, for those who are willing to ponder on their own the difference between the motion types, a hint: once a continuous and uninterruptible motion is started (by the beetle) in both cases - translational and rotational - what can be said about the ability of a system to bring itself into its initial position using internal forces only?
|
|
 IP Logged IP Logged |
|
|
|
|
 WRITE MATH!
WRITE MATH!
 Home
Home  Help
Help  Search
Search  Members
Members  Login
Login  Register
Register WRITE MATH!
WRITE MATH!
 Home
Home  Help
Help  Search
Search  Members
Members  Login
Login  Register
Register