Author |
Topic: Two Inscribed Circles (Read 1865 times) |
|
Barukh
Uberpuzzler
Gender: 
Posts: 2276
|
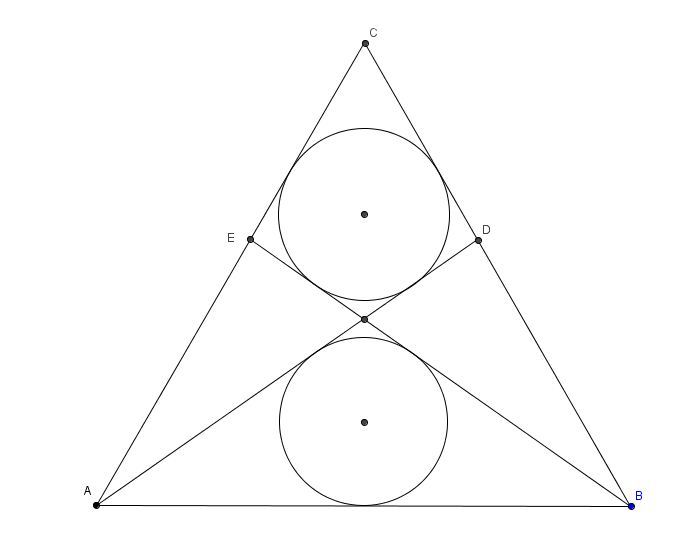
As this problem generated much interest, I decided to post another one - this time more difficult.
Triangle ABC is equilateral with side 1. Both inscribed circles have the same radius r.
Find r. As before, the preference is for simple and elegant solutions (let's see if tiling works here).
|
| « Last Edit: Jul 29th, 2014, 11:02pm by Barukh » |
 IP Logged |
|
|
|
towr
wu::riddles Moderator
Uberpuzzler
Some people are average, some are just mean.
Gender:
Posts: 13730
|
 |
Re: Two Inscribed Circles
« Reply #1 on: Jul 30th, 2014, 8:55am » |
 Quote  Modify
|
Seems to be about 1/(2 pi)
[edit]Close but no cigar[/edit]
|
| « Last Edit: Jul 30th, 2014, 9:54am by towr » |
IP Logged |
Wikipedia, Google, Mathworld, Integer sequence DB
|
|
|
rloginunix
Uberpuzzler
Posts: 1029
|
|
Re: Two Inscribed Circles
« Reply #2 on: Jul 30th, 2014, 9:46am » |
Quote Modify
|
I don't have a solution yet. Just a small observation of dynamic nature.
Imagine E and D sliding along CA and CB. When E and D get closer to A and B the bottom circle diminishes while the top one grows. The degenerate case being R(bottom) = 0 and R(top) = R(Incircle) (when E = A, D = B).
When E and D get closer to C the inverse happens - the bottom circle grows while the top one diminishes. The degenerate case being R(bottom) = R(Incircle) and R(top) = 0 (when E = D = C).
I don't remember the exact name but, as I recall, there is a theorem in math analysis that deals with the intermediate values of a contiguous function. Since the above movement is contiguous, based on that theorem we can conclude that there must exist such a state when R(bottom) = R(top).
I think a key to find a solution to this problem is understanding what that condition is. I would even think that there should be a ruler and compass construction - Barukh made the drawing somehow.
|
|
IP Logged |
|
|
|
towr
wu::riddles Moderator
Uberpuzzler
Some people are average, some are just mean.
Gender:
Posts: 13730
|
r = (sqrt(3)-sqrt(2))/2
(for solution see attachment)
|
| « Last Edit: Jul 30th, 2014, 11:41am by towr » |
IP Logged |
Wikipedia, Google, Mathworld, Integer sequence DB
|
|
|
rloginunix
Uberpuzzler
Posts: 1029
|
|
Re: Two Inscribed Circles
« Reply #4 on: Jul 30th, 2014, 10:51am » |
Quote Modify
|
If it's a solution - I don't want to look. If it's a hint for my post - I may. I just want to know which one is it? Thanks.
|
|
IP Logged |
|
|
|
towr
wu::riddles Moderator
Uberpuzzler
Some people are average, some are just mean.
Gender:
Posts: 13730
|
|
Re: Two Inscribed Circles
« Reply #5 on: Jul 30th, 2014, 11:46am » |
Quote Modify
|
The attachment is a solution (or a pretty good indication of one).
The hidden text is the answer I got.
Guess it was a good choice to attach it as pdf, so that the image wasn't displayed and possibly gave away spoilers.
There's very likely a nicer/simpler solution, so I'll keep looking as well.
|
|
IP Logged |
Wikipedia, Google, Mathworld, Integer sequence DB
|
|
|
dudiobugtron
Uberpuzzler
Posts: 735
|
|
Re: Two Inscribed Circles
« Reply #6 on: Jul 30th, 2014, 6:10pm » |
Quote Modify
|
@ rloginunix:
The name of the theorem is pretty boring, it's called the 'Intermediate Value Theorem'.
Edit:
I wanted to solve it for myself before seeing how it was done diagrammatically. Once I had gotten the same answer as towr, I looked at towr's working to see how it was actually supposed to be done. I have a couple of questions about that method to try and understand how it worked.
Questions:
Actually just one question - how did you get your BE = 2r? I know how I worked it out (laboriously...), but I can't see yet how it follows from your diagrams.
Thanks heaps for your help in understanding it!
-------
My own (excessively inelegant) method was to (using the original labels, not towr's) use coordinate geometry. I set A = (0,0), and then solved the equations for the bottom circle with AD, and the top circle with AC, to find the values which gave 1 point of intersection. Finding the gradient of AD gave me the height of the intersection point between AD and BE in terms of r; altogether the solutions gave me a (cubic) equation for the height of the triangle in terms of r, which I then cheatingly solved using wolfram alpha... I can post the full working if anyone is interested, but it's obviously not a good way to solve it!
|
| « Last Edit: Jul 30th, 2014, 7:28pm by dudiobugtron » |
IP Logged |
|
|
|
towr
wu::riddles Moderator
Uberpuzzler
Some people are average, some are just mean.
Gender:
Posts: 13730
|
|
Re: Two Inscribed Circles
« Reply #7 on: Jul 30th, 2014, 10:11pm » |
Quote Modify
|
on Jul 30th, 2014, 6:10pm, dudiobugtron wrote:Questions:
Actually just one question - how did you get your BE = 2r? I know how I worked it out (laboriously...), but I can't see yet how it follows from your diagrams. |
|
Because we're dealing with an equilateral triangle, we can put a circle with radius r at B that touches the circle centered on E. Which is easiest to see by imagining you can make a hexagon of copies of the triangle and realizing you can fit a same-sized circle in a hexagon of circles.
|
| « Last Edit: Jul 30th, 2014, 10:13pm by towr » |
IP Logged |
Wikipedia, Google, Mathworld, Integer sequence DB
|
|
|
dudiobugtron
Uberpuzzler
Posts: 735
|
|
Re: Two Inscribed Circles
« Reply #8 on: Jul 31st, 2014, 12:19am » |
Quote Modify
|
It took me a while even with your explanation, but I get it pretty well now - thanks towr! 
|
|
IP Logged |
|
|
|
Barukh
Uberpuzzler
Gender:
Posts: 2276
|
|
Re: Two Inscribed Circles
« Reply #9 on: Jul 31st, 2014, 8:37am » |
Quote Modify
|
on Jul 30th, 2014, 10:11pm, towr wrote:
Because we're dealing with an equilateral triangle, we can put a circle with radius r at B that touches the circle centered on E. Which is easiest to see by imagining you can make a hexagon of copies of the triangle and realizing you can fit a same-sized circle in a hexagon of circles. |
|
Isn't it just 30-60-90 triangle?
|
|
IP Logged |
|
|
|
towr
wu::riddles Moderator
Uberpuzzler
Some people are average, some are just mean.
Gender:
Posts: 13730
|
|
Re: Two Inscribed Circles
« Reply #10 on: Jul 31st, 2014, 8:45am » |
Quote Modify
|
There's also that, yes. It's equivalent, but the visual explanation is what gave me my "duh, obviously"-erlebniss.
|
|
IP Logged |
Wikipedia, Google, Mathworld, Integer sequence DB
|
|
|
towr
wu::riddles Moderator
Uberpuzzler
Some people are average, some are just mean.
Gender:
Posts: 13730
|
I found a seemingly nice way to construct/draw the problem, but I'm still missing a simple proof that the large circle centered on F touches the small circle(s) at H.
(Or, actually, the way I constructed it, that AD touches the two small circles in the triangle. But that was a given in the problem.)
|
| « Last Edit: Jul 31st, 2014, 12:01pm by towr » |
IP Logged |
Wikipedia, Google, Mathworld, Integer sequence DB
|
|
|
dudiobugtron
Uberpuzzler
Posts: 735
|
|
Re: Two Inscribed Circles
« Reply #12 on: Jul 31st, 2014, 6:13pm » |
Quote Modify
|
You could work out the the distance from H to GC (call this distance HI), and then check that 3 * HI + 2 *HC = FG
Not sure if that's any simpler than your original proof though!
|
| « Last Edit: Jul 31st, 2014, 6:13pm by dudiobugtron » |
IP Logged |
|
|
|
rloginunix
Uberpuzzler
Posts: 1029
|
|
Re: Two Inscribed Circles
« Reply #13 on: Jul 31st, 2014, 9:05pm » |
Quote Modify
|
After much deliberation I think I got it. This problem did send me for a loop. AELDBL being a butterfly, at first I thought the butterfly theorem will work. Then, AD being a nice cevian, I thought "Stewart's theorem"? But I introduced four variables and was only able to come up with one equation ...
Then, looking back at my third post in the original Barukh's "Inscribed Circle" problem I eventually realized that 1). the drawings look very similar, 2). I've done this before, 3). I'm staring at a potential solution. And a potential solution is staring back at me - the "square areas method" if you will. I called it not elegant back then and so be it now, at least I think I've found a solution and the chase for simplicity and elegance can begin.
Notation: S(ABC) = square area of a triangle ABC. For ease of reference I put the 90-degree vertex in the triangle names below in dead center.
This is what I call a Division approach: divide the whole into parts so simple that each and every one can be solved on its own. Find a way to combine the solutions of the parts into a solution of the whole. This idea was used successfully to invent calculus but here the things are much simpler: the whole is the entire parent triangle and the parts are its smaller right triangles.
Due to obvious symmetry the square area of the right triangle on the "right" must be equal to the square area of the right triangle on the "left":
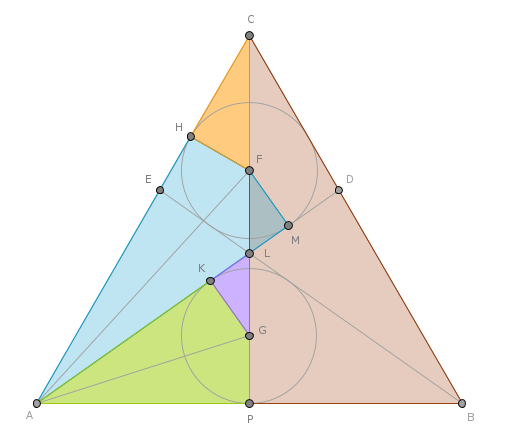
S(CPB) = S(CPA) = 2S(GPA) + S(GKL) + 2S(AHF) - S(FML) + S(CHF)
but S(GKL) = S(FML) - the angles at L being vertical and tangents LK and LM to circles of equal radii GK and FM being equal in length (SAS by construction). So these two square areas cancel each other out:
S(CPA) = 2S(GPA) + 2S(AHE) + S(CHF)
1/2*1/2*sqrt(3)/2 = 2*1/2*1/2*r + 2*1/2*AH*r + 1/2*CH*r
From the 30-60-90 triangle CHF it follows that CH = r*ctg(30) = r*sqrt(3).
AH = AC - CH = 1 - r*sqrt(3) and hence:
sqrt(3)/8 = r/2 + r(1 - sqrt(3)) + r*sqrt(3)/2
Multiply both sides by 8 and after some rearrangements we get the quadratic equation:
4sqrt(3)r^2 - 12r + sqrt(3) = 0
Divide both sides by 4sqrt(3):
r^2 - sqrt(3)r + 1/4 = 0
Solving it using the well known formula of (-b +- sqrt(b^2 - 4ac))/2 we get:
r(1,2) = (sqrt(3) +- sqrt(2))/2
The first root must be thrown out - r can't be greater than 1 or the side of the triangle. Hence, r = (sqrt(3) - sqrt(2))/2. Which, according to OpenSuse's calculator, is 0.158918623.
P.S.
Again, I didn't look at towr's idea. Let me know if mine is a duplicate.
|
|
IP Logged |
|
|
|
rloginunix
Uberpuzzler
Posts: 1029
|
|
Re: Two Inscribed Circles
« Reply #14 on: Jul 31st, 2014, 9:16pm » |
Quote Modify
|
Dang it. I now see I violated Barukh's "no trig" requirement with ctg(30). Sorry. It's in the bones. OK, so triangle CHF is 30-60-90 so FH = r and CF = 2r. From Pythagoras it follows that CH = r*sqrt(3).
|
|
IP Logged |
|
|
|
Barukh
Uberpuzzler
Gender:
Posts: 2276
|
|
Re: Two Inscribed Circles
« Reply #15 on: Aug 1st, 2014, 3:29am » |
Quote Modify
|
Nice solutions, towr and rloginunix!
The solution I had in mind was actually rloginunix's, but I think towr's is simpler and more elegant.
on Jul 31st, 2014, 11:54am, towr wrote:| I found a seemingly nice way to construct/draw the problem |
|
Once you know the answer - the construction is straightforward.
Quote:| ...but I'm still missing a simple proof that the large circle centered on F touches the small circle(s) at H. |
|
This is a different matter! Looks like you are trying to find another solution?
|
|
IP Logged |
|
|
|
SWF
Uberpuzzler
Posts: 879
|
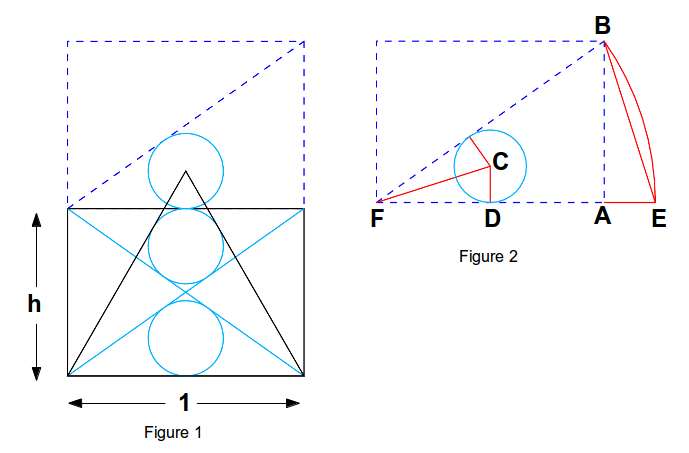
First a couple direct solutions, then next post has a different geometric interpretation:
Draw the 1 by h rectangle around the inscribed circles as shown in Figure 1, and a duplicate above it (dotted lines). Because of equilateral triangle, radius of inscribed circles, r = sqrt(3)/2 - h = CD. Construct the red lines on the upper rectangle (Figure 2). Triangle FBE is isoceles, and triangle FCD is similar to BEA:
CD/FD = AE/AB = (sqrt(3)/2-h)/(0.5) = (FB-AF)/AB = (sqrt(1+h2)-1)/h
Solve the above for h. A shortcut to minimize algebra is to write as:
1+h2-sqrt(1+h2) = 3h2-sqrt(3h2)
and note that both sides are the same function with different expression as the argument f(1+h2)=f(3h2)
so one solution is 1+h2 = 3h2,
h= sqrt(2)/2 (discarding the negative solution, and the easy-to-guess h=0 solution).
r = sqrt(3)/2 - h = sqrt(3)/2 - sqrt(2)/2
A second method that is more direct (without need of the extra construction lines), use the trig identity:
tan(2x)=2*tan(x)/(1-tan2(x))
Angle AFB is twice angle DFC. tan(AFB)=h tan(DFC)=r/0.5=2r=2(sqrt(3)/2-h)=sqrt(3)-2h giving:
h=2*(sqrt(3)-2h)/(1-(sqrt(3)-2h)2)
h*(2-4*h2)=sqrt(3)*(2-4*h2)
Either h=sqrt(3) or (2-4*h2)=0 (i.e. h=sqrt(2)/2 or -sqrt(2)/2 or sqrt(3) ) h=sqrt(2)/2 is clearly the one we are looking for.
|
|
IP Logged |
|
|
|
SWF
Uberpuzzler
Posts: 879
|
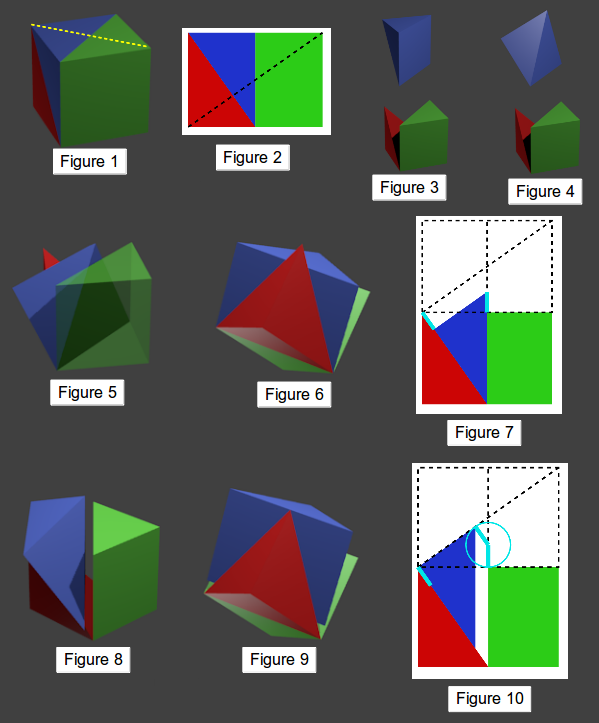
A geometrical interpretation is to look at a cube that is sqrt(2)/2 on each side, and cut it into 3 pieces (Figure 1). The cut between red and blue pieces forms an equilateral triangle of side 1, and the cut between blue and green pieces makes a 1 by sqrt(2)/2 rectangle. The yellow dotted line shows a cutting plane that is used for the cross-sectional views of Figures 2, 7, and 10.
Figure 2 is a cross section of the cube. Note that the black dotted line (cube body diagonal) is normal to the boundary between the red and blue regions (by symmetry of the red piece). In Figure 3, the blue volume was removed, then reoriented in Figure 4.
The reoriented blue piece was reinserted in Figure 5. Interface between blue and green pieces is the triangle against the 1 by sqrt(2)/2 rectangle- just like the shape shown in my previous post. Figure 6 shows it from another angle, with boundary between red and blue also being a combination of triangle and rectangle. Figure 7 is a section through the new arragement of pieces with dotted lines for a duplicate cube stacked on top with body diagonal added. The two cyan lines are the same length: equal to the difference between heights of the equilateral triangle and the rectangle.
Next slide the blue piece up to the tip of the red piece (Figures 8 and 9). The distance slid is equal to the length of the cyan lines, and since the interface between red and blue pieces is normal to the dotted black line of Figure 9, the upper tip of the blue piece traces a segment normal to dotted line and that segment has same length as the other cyan lines. Those cyan lines define a circle tangent to the required lines. Radius of that circle is difference between height of equilaterial triangle and height of cube: r=sqrt(3)/2-sqrt(2)/2.
|
|
IP Logged |
|
|
|
SWF
Uberpuzzler
Posts: 879
|
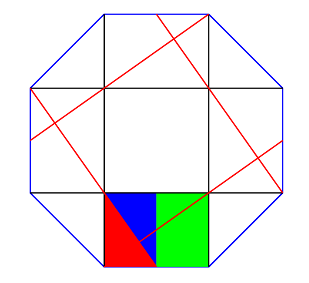
Another thing I would like to comment on is that the geometry of a regular octagon of side length 1 can be used for a 2-D version of the cube interpretation presented above. The red, blue and green pieces shown below correspond to the cross-section pictures in my previous post. The four red lines show how the boundary between red and blue areas is normal to the diagonal of the 1 by sqrt(2)/2 rectangle. That the length of the boundary between red and blue equals height of equilateral triangle can be seen from Pythagorean Theorem.
Although the cube interpretation is maybe tougher to visualize, I like it better because of the way the equilateral triangle appears, rather than verifying the diagonal has height of the triangle through Pythagorean theorem.
|
|
IP Logged |
|
|
|
rloginunix
Uberpuzzler
Posts: 1029
|
|
Re: Two Inscribed Circles
« Reply #19 on: Oct 15th, 2014, 10:14am » |
Quote Modify
|
That is absolutely awesomely cool. Especially the switch to 3D. I wonder what was the chain of logical steps to start with a triangle and end up with a 3D solid. I also wonder why Justin Bieber and the Kardashians make the news and this does not.
If you do not mind, what is the software you use to render the 3D solids? OpenSCAD? Thanks in advance.
|
|
IP Logged |
|
|
|
SWF
Uberpuzzler
Posts: 879
|
|
Re: Two Inscribed Circles
« Reply #20 on: Oct 16th, 2014, 10:27pm » |
Quote Modify
|
The 1 by sqrt(2)/2 rectangle turned out to have quite a few unique properties, sort of like working with the Golden Ratio.
The 3-D pictures were made with Blender.
|
|
IP Logged |
|
|
|
|
RIDDLES SITE
WRITE MATH!
Home 
Help 
Search 
Members 
Login 
Register
Reply
Notify of replies
Send Topic
Print
twoinscribedcircles.pdf