 Author
Author |
Topic: Char poly of binomial matrix (Read 3356 times) |
|
Eigenray
wu::riddles Moderator
Uberpuzzler
    

Gender: 
Posts: 1948
|
 |
Re: Char poly of binomial matrix
« Reply #25 on: Mar 22nd, 2007, 11:34am » |
 Quote Quote  Modify Modify
|
on Mar 22nd, 2007, 6:47am, Barukh wrote:| That doesn't help to find the multiplicites in our case, does it? |
|
I'm not sure what you mean: we know the minimal polynomial of A divides t3-1, so it has distinct roots. This implies all Jordan blocks of A are 1x1, i.e., A is diagonalizable. The multiplicity of an eigenvalue in the characteristic polynomial is the sum of the sizes of the Jordan blocks corresponding to it, but this is just the number of times it appears in the diagonalization of A.
Quote:
Another equation would be b = b’ (because  , ,  2 are kind of conjugate) 2 are kind of conjugate) |
|
This reasoning would be enough in the case p=2 mod 3, because then   Fp, so the Frobenius automorphism fixes Fp (hence the characteristic polynomial) but interchanges Fp, so the Frobenius automorphism fixes Fp (hence the characteristic polynomial) but interchanges  and and  2. 2.
But in the case p=1 mod 3, a different argument is needed. For example, there are matrices with entries in F7, with eigenvalues 1, 2, 4, of arbitrary multiplicities.
Quote:, and the last is: a-b = Tr(A) mod p. The latter turns out to be equal to -(-1)p mod 3. This gives the answer.
It remains then to prove the last identity.
|
|
Exactly. We have of course
Tr(A) =  k=0p-1 C(2k, k). k=0p-1 C(2k, k).
As a sort of hint, what is  k=0 k=0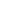 C(2k, k) xk? Why? C(2k, k) xk? Why?
|
|
 IP Logged IP Logged |
|
|
|
Barukh
Uberpuzzler
    

Gender: 
Posts: 2276
|
 |
Re: Char poly of binomial matrix
« Reply #26 on: Mar 23rd, 2007, 8:25am » |
 Quote Quote  Modify Modify
|
on Mar 22nd, 2007, 11:34am, Eigenray wrote:
I'm not sure what you mean: we know the minimal polynomial of A divides t3-1, so it has distinct roots. |
|
I meant that using all this information didn't show (at least to me) the way to calculate the multiplicites.
Quote:| This implies all Jordan blocks of A are 1x1, i.e., A is diagonalizable. |
|
This takes me once again to the point we were discussing earlier and that is still not completely clear for me (maybe, I simply misunderstand the concept of diagonalization?).
Take p = 5. The eigenvalues of A(5) will be, as we know, 1,  , ,  , ,  2, 2,  2, and these same values will be at the diagonal of the diagonalization of A. Now, 4 out of 5 values are not in Z/5. This clear is not in line with you statement earlier in this thread: 2, and these same values will be at the diagonal of the diagonalization of A. Now, 4 out of 5 values are not in Z/5. This clear is not in line with you statement earlier in this thread:
Quote:| that a matrix is diagonalizable (over some field F) iff its minimal polynomial splits into distinct linear factors over F. |
|
But later you mentioned that “over F” should be replaced by “over some extension of F”. Which extension of Z/5 can we take in this case?
Quote:| But in the case p=1 mod 3, a different argument is needed. For example, there are matrices with entries in F7, with eigenvalues 1, 2, 4, of arbitrary multilicities. |
|
Yes… I felt something is missing here.
Quote:As a sort of hint, what is  k=0 k=0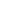 C(2k, k) xk? Why? C(2k, k) xk? Why? |
|
It equals (1-4x)-1/2 (because of a nice identity involving convolution of central binomial coefficients).

|
| « Last Edit: Mar 23rd, 2007, 8:27am by Barukh » |
 IP Logged IP Logged |
|
|
|
Eigenray
wu::riddles Moderator
Uberpuzzler
    

Gender: 
Posts: 1948
|
 |
Re: Char poly of binomial matrix
« Reply #27 on: Mar 23rd, 2007, 1:36pm » |
 Quote Quote  Modify Modify
|
on Mar 23rd, 2007, 8:25am, Barukh wrote:
I meant that using all this information didn't show (at least to me) the way to calculate the multiplicites. |
|
It wasn't really intended to, it was just to show how the minimal polynomial can be read off from the Jordan normal form; in this case, knowing the minimal polynomial gives you information about the Jordan normal form.
Quote:Take p = 5. The eigenvalues of A(5) will be, as we know, 1,  , ,  , ,  2, 2,  2, and these same values will be at the diagonal of the diagonalization of A. Now, 4 out of 5 values are not in Z/5. 2, and these same values will be at the diagonal of the diagonalization of A. Now, 4 out of 5 values are not in Z/5. |
|
So A(5) is not diagonalizable over F5=Z/5. But it is diagonalizable over F25 = F5[ ], a degree 2 extension of F5, obtained by adjoining ], a degree 2 extension of F5, obtained by adjoining  , a solution to x2+x+1=0. That is, F25 is isomorphic to F5[x]/(x2+x+1), where , a solution to x2+x+1=0. That is, F25 is isomorphic to F5[x]/(x2+x+1), where  corresponds to the equivalence class of x. corresponds to the equivalence class of x.
Explicitly, over this field, a basis of eigenvectors is
(1, 3, 3, 3, 1),
(1, 1, -1+2 , 0, , 0,  2) 2)
(0, 1, -2- , 1+ , 1+ , 0) , 0)
(1, 1, -1+2 2, 0, 2, 0,  ) )
(0, 1, -2- 2, 1+ 2, 1+ 2, 0), 2, 0),
with eigenvalues 1,  , ,  , ,  2, 2,  2, respectively. 2, respectively.
Quote:This clear is not in line with you statement earlier in this thread:
a matrix is diagonalizable (over some field F) iff its minimal polynomial splits into distinct linear factors over F |
|
That statement is true. Over F5, the minimal polynomial factors into irreducibles as (x-1)(x2+x+1). It doesn't split into linear factors, but it's still separable, because over the field F5[ ], it factors into distinct linear factors (x-1)(x- ], it factors into distinct linear factors (x-1)(x- )(x- )(x- 2). So the matrix is diagonalizable over F5[ 2). So the matrix is diagonalizable over F5[ ], but not over F5. ], but not over F5.
Quote:| But later you mentioned that “over F” should be replaced by “over some extension of F”. |
|
When I said that I was referring to
Quote:x3-1, which has distinct roots (of degree no more than 2) over Fp iff p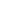 3 3 |
|
What I meant was, if you consider x3-1 as a polynomial, not over Q, but with coefficients in Fp, then it "has distinct roots" iff p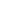 3. And when I say "has distinct roots," I mean it's separable: it splits into distinct linear factors over some extension of the field containing its coefficients. In this case, the splitting field has degree 1 or 2 over Fp. 3. And when I say "has distinct roots," I mean it's separable: it splits into distinct linear factors over some extension of the field containing its coefficients. In this case, the splitting field has degree 1 or 2 over Fp.
I guess I should have used the term "separable" because it depends only on the polynomial (compared to the question "does f have a root", which depends on the field you're working in). That is, if f has coefficients in some field F 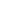 K, then f is separable, viewed as a polynomial over F, iff it's separable as a polynomial over K. K, then f is separable, viewed as a polynomial over F, iff it's separable as a polynomial over K.
(Although the term separable is also used to mean only that each irreducible factor has distinct roots in some extension -- compare planetmath to wikipedia -- and with this usage, it does depend on the field.)
Quote:| Yes… I felt something is missing here. |
|
What do you know about A and A-1?
Quote:| It equals (1-4x)-1/2 (because of a nice identity involving convolution of central binomial coefficients). |
|
The other way to think of it is because
(1-4x)-1/2 =  C(-1/2, k) (-4x)k = C(-1/2, k) (-4x)k =  C(2k, k) xk C(2k, k) xk
|
| « Last Edit: Mar 23rd, 2007, 2:03pm by Eigenray » |
 IP Logged IP Logged |
|
|
|
Barukh
Uberpuzzler
    

Gender: 
Posts: 2276
|
 |
Re: Char poly of binomial matrix
« Reply #28 on: Mar 25th, 2007, 6:24am » |
 Quote Quote  Modify Modify
|
Quote:The other way to think of it is because
(1-4x)-1/2 =  C(-1/2, k) (-4x)k = C(-1/2, k) (-4x)k =  C(2k, k) xk C(2k, k) xk |
|
Oh, I see. That’s a very simple demonstration. Here’s how I did it (actually, using the approach taken in the aforementioned paper).
I started from the following identity:
1x3x…(2k-1) = 2-k(k+1)(k+2)…(2k)
which is simply demonstrated by induction. Now, replace every term i at the left with (p-i), and take modulo p:
(p-1)(p-3)…(p-2k+1) = (-2)-k(k+1)(k+2)…(2k) mod p
Divide both sides by k!2k to get
C((p-1)/2, k) = C(2k, k)(-4)-k mod p.
Therefore (the summation is from 0 to (p-1)/2):
Tr(A) =  C(2k,k) = C(2k,k) =  C((p-1)/2, k)(-4)k = (binom!) = (-3) (p-1)/2 mod p C((p-1)/2, k)(-4)k = (binom!) = (-3) (p-1)/2 mod p
Using the results from quadratic residues theory, we obtain:
(-3) (p-1)/2 mod p = (-3 | p) = (p | -3) = -(-1)p mod 3,
where (x|y) is Legendre symbol.
Eigenray, I apologize for this lengthy demonstration, yours is much more concise, but I thought that way more people will follow the discussion.
I must say that I really enjoyed working on this problem!
|
| « Last Edit: Mar 25th, 2007, 6:39am by Barukh » |
 IP Logged IP Logged |
|
|
|
Eigenray
wu::riddles Moderator
Uberpuzzler
    

Gender: 
Posts: 1948
|
 |
Re: Char poly of binomial matrix
« Reply #29 on: Mar 25th, 2007, 2:42pm » |
 Quote Quote  Modify Modify
|
on Mar 25th, 2007, 6:24am, Barukh wrote:| Using the results from quadratic residues theory, we obtain |
|
We don't actually need quadratic reciprocity here (or rather, the special case we need has a short proof). For p > 3 an odd prime, -3 is a square mod p iff the equation x2 + x + 1 = 0 has a solution in Fp (since (2x+1)2 = -3). But any solution will be a primitive 3rd root of unity; since Fp* is cyclic, this exists iff p = 1 mod 3. So (-3|p) = (p|3).
Quote:| I must say that I really enjoyed working on this problem! |
|
And thank you for working on it. But it's not done yet! So, what is the relation between A(p) and A(pk), that led me to this question in the first place?
|
|
 IP Logged IP Logged |
|
|
|
Barukh
Uberpuzzler
    

Gender: 
Posts: 2276
|
 |
Re: Char poly of binomial matrix
« Reply #30 on: Mar 29th, 2007, 10:10am » |
 Quote Quote  Modify Modify
|
on Mar 25th, 2007, 2:42pm, Eigenray wrote:| But it's not done yet! So, what is the relation between A(p) and A(pk), that led me to this question in the first place? |
|
Maybe, the following is relevant (assuming k =2 for a moment) :
From the previously mentioned property, it follows that
Amp+i,np+q(p2) = Amn(p) Aij(p)
Putting it differently, A(p2) may be divided into p2 blocks Bij of size pxp each, so that Bij = A(p)Aij(p). I learned just today that this is called matrix direct, or Kronecker product of matrix A(p) with itself. The resulting matrix has eigenvalues equal to the Cartesian product of eigenvalues of the base matrices. In our case, eigenvalues of A(p) form a group under multiplication modulo p, therefore A(p2) will have the same eigenvalues. Their multiplicities are easily computed.
This is easily extended to any natural k.
|
|
 IP Logged IP Logged |
|
|
|
Eigenray
wu::riddles Moderator
Uberpuzzler
    

Gender: 
Posts: 1948
|
 |
Re: Char poly of binomial matrix
« Reply #31 on: Mar 30th, 2007, 7:07pm » |
 Quote Quote  Modify Modify
|
on Mar 29th, 2007, 10:10am, Barukh wrote:| Putting it differently, A(p2) may be divided into p2 blocks Bij of size pxp each, so that Bij = A(p)Aij(p). |
|
Yes, precisely. When p=2, this says that if A=A(q), then A(2q) is
[ A A ]
[ A O ],
which is exactly how Sierpinski's triangle is formed. (In fact, in this case [A(2k) mod 2] and [A(2) mod 2]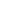 ... ...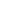 [A(2) mod 2] are equal as integer matrices, not just mod 2.) [A(2) mod 2] are equal as integer matrices, not just mod 2.)
Quote:| I learned just today that this is called matrix direct, or Kronecker product of matrix A(p) with itself. The resulting matrix has eigenvalues equal to the Cartesian product of eigenvalues of the base matrices. |
|
Moreover, if v, w, are eigenvectors of A, B, with eigenvalues  , ,  , respectively, then v , respectively, then v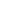 w is an eigenvector of A w is an eigenvector of A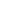 B with eigenvalue B with eigenvalue   . And if {vi}, {wj} are bases of V, W, respectively, then {vi . And if {vi}, {wj} are bases of V, W, respectively, then {vi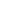 wj} is a basis of V wj} is a basis of V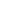 W. So we can even give an eigenbasis for A(pk) (for p W. So we can even give an eigenbasis for A(pk) (for p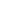 3). 3).
Another way to see this: if UAU-1, VBV-1 are diagonal (or at least upper-triangular), then (U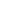 V)(A V)(A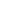 B)(U B)(U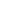 V)-1 = (UAU-1) V)-1 = (UAU-1)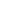 (VBV-1) is also diagonal (or upper-triangular -- so we can still read off the eigenvalues). (VBV-1) is also diagonal (or upper-triangular -- so we can still read off the eigenvalues).
|
|
 IP Logged IP Logged |
|
|
|
|
 WRITE MATH!
WRITE MATH!
 Home
Home  Help
Help  Search
Search  Members
Members  Login
Login  Register
Register WRITE MATH!
WRITE MATH!
 Home
Home  Help
Help  Search
Search  Members
Members  Login
Login  Register
Register