| relatively easy |
| MARBLE JARS I |
you are a prisoner in a foreign land. your fate will be determined by a little game. there are two jars, one with 50 white marbles, and one with 50 black marbles. at this point, you are allowed to redistribute the marbles however you wish (e.g. swap a black marble with a white marble, etc.): the only requirement is that after you are done with the redistribution, every marble must be in one of the two jars. afterwards, both jars will be shaken up, and you will be blindfolded and presented with one of the jars at random. then you pick one marble out of the jar given to you. if the marble you pull out is white, you live; if black, you die. how should you redistribute the marbles to maximize the probability that you live; what is this maximum probability (roughly)?
Hint: Redundancy.
|
| ARAB SHEIKH CAMELS |
An Arab sheikh is old and must will his fortune to one of his two sons. He makes a
proposition. His two sons will ride their camels in a race, and whichever camel crosses
the finish line last will win the fortune for its owner. During the race, the two
brothers wander aimlessly for days, neither willing to cross the finish line. In
desperation, they ask a wise man for advice. He tells them something; then the brothers
leap onto the camels and charge toward the finish line. What did the wise man say?
|
| 3 HATS |
There are 3 black hats and 2 white hats in a box. Three men (we will call them
A, B, & C) each reach into the box and place one of the hats on his own head.
They cannot see what color hat they have chosen. The men are situated in a way
that A can see the hats on B & C's heads, B can only see the hat on C's head
and C cannot see any hats.
When A is asked if he knows the color of the hat he is wearing, he says no.
When B is asked if he knows the color of the hat he is wearing he says no.
When C is asked if he knows the color of the hat he is wearing he says yes and
he is correct.
What color hat and how can this be?
|
| HUMMINGBIRD |
One train leaves Los Angeles at 15mph heading for New York. Another train leaves from New
York at 20mph heading for Los Angeles on the same track. The distance between LA and NY is about 5000 miles.
If a bird, flying at 25mph, leaves from Los Angeles at the same time as the train and flies back and forth between
the two trains until they collide, how far will the bird have traveled?
|
| FOOT SIZE IMPLIES SPELLING ABILITY |
scientific studies have shown that there is a direct, positive correlation between foot
size and performance in spelling bees / spelling tests. how can you explain this correlation?
Hint: Don't think too hard ... it's just for the most obvious reasons.
|
| NONHOMOGENEOUS ROPE BURNING |
you have two ropes, each of which takes one hour to burn completely. both of these ropes are
nonhomogeneous in thickness, meaning that some parts of the ropes are chunkier than other parts
of the rope. using these nonhomogeneous ropes and a lighter, time 45 minutes.
Note: Some clarification on what is meant by nonhomogeneous. For instance, maybe a particular section of
rope that is 1/8 of the total length is really chunky, and takes 50 minutes to burn off. then it would take 10 minutes to
burn off the remaning 7/8, since we know that the whole rope takes an hour to burn off. that's just an example; we don't know any such ratios beforehand. The point is, if you look at one of your ropes and cut it into pieces, you have no clue how long any individual piece will take to burn off.
|
WILLYWUTANG AND
THE BURNING ISLAND OF DOOM |
willywutang is hanging out on a heavily forested island that's really narrow: it's a narrow strip of land that's ten
miles long. let's label one end of the strip A, and the other end B. a fire has started at A, and the fire is moving
toward B at the rate of 1 mph. at the same time, there's a 2 mph wind blowing in the direction from A toward B. what
can willywu do to save himself from burning to death?! assume that willywu can't swim and there are no boats, jetcopters,
teleportation devices, etc.. (if he does nothing, willywu will be toast after at most 10 hours, since 10 miles / 1 mph = 10 hours)
Forum thread: click here
|
| TWO COIN FLIPS |
i flip a penny and a dime and hide the result from you. "at least one of the coins came up heads", i announce. what is the chance that both coins came up heads?
Hint: Think again; conditional probability is often very nonintuitive. Write out a table of possibilities.
|
| COIN MACHINE WEIGHING |
you have 20 coin machines, each of which produce the same kind of coin. you know how much a coin is supposed to weigh. one of the machines is defective, in that every coin it produces weighs 1 ounce less than it is supposed to. you also have an electronic weighing machine. how can you determine which of the 20 machines is defective with only one weighing? (by one use, we mean you put a bunch of stuff on the machine and read a number, and that's it -- you not allowed to accumulate weight onto the machine and watch the numbers ascend, because that's just like multiple weighings). you are allowed to crank out as many coins from each machine as you like.
|
| HOURGLASSES |
You have two hourglasses: a 7 minute one and an 11 minute one. Using just these hourglasses, accurately time 15 minutes.
|
| LOGICAL SIGNS I |
You are an archaeologist that has just unearthed a long-sought pair of ancient treasure chests. One chest is plated with silver, and the other is plated with gold. According to legend, one of the two chests is filled with great treasure, whereas the other chest houses a man-eating python that can rip your head off. Faced with a dilemma, you then notice that there are inscriptions on the chests:
| Silver Chest | Gold Chest |
| This chest contains the python. | One of these two inscriptions is true. |
Based on these inscriptions, which chest should you open?
Hint: argumentum ad ignorantiam. Thanks to Peter Surda for e-mailing me his unconventional analysis.
|
| LOGICAL SIGNS II |
You are an archaeologist that has just unearthed a long-sought triplet of ancient treasure chests. One chest is plated with silver, one with gold, and one with bronze. According to legend, one of the three chests is filled with great treasure, whereas the other two chests both house man-eating pythons that can rip your head off. Faced with a dilemma, you then notice that there are inscriptions on the chests:
| Silver Chest |
Gold Chest |
Bronze Chest |
| Treasure is in this Chest. |
Treasure is not in this Chest. |
Treasure is not in the Gold Chest. |
You know that at least one of the inscriptions is true, and at least one of the inscriptions is false. Which chest do you open?
|
CHESS PUZZLE I
C |
Green numbers indicate how many pieces could move to that square on the next move. Blue squares show the possible locations of the following five different chess pieces:
How are the five pieces arranged?
|
CHESS PUZZLE II
C |
Green numbers indicate how many pieces could move to that square on the next move. Blue squares show the possible locations of the following five different chess pieces:
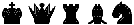
How are the five pieces arranged?
|
| CORK, BOTTLE, COIN |
If you were to put a coin into an empty bottle and then insert a cork in the bottle's opening, how could you remove the coin without taking out the cork or breaking the bottle?
Hint: Actually, people solve this riddle everyday. Let's say you're opening a wine bottle, and in the process you break the cork. What's the only thing left to do?
|
| FAMILY RELATIONS |
Speaker: "Brothers and Sisters, I have none. But this man's Father is my Father's son."
Who is the speaker talking about?
|
| ANALOG CLOCK I |
An analog clock reads 3:15. What is the angle between the minute hand and hour hand?
|
| ANALOG CLOCK II |
Imagine an analog clock set to 12 o'clock. Note that the hour and minute hands overlap.
How many times each day do both the hour and minute hands overlap? How would you
determine the exact times of the day that this occurs?
|
| APPLES AND ORANGES |
There are three closed and opaque cardboard boxes. One is labeled "APPLES", another is labeled "ORANGES", and the last is labeled "APPLES AND ORANGES". You know that the labels are currently misarranged, such that no box is correctly labeled. You would like to correctly rearrange these labels. To accomplish this, you may draw only one fruit from one of the boxes. Which box do you choose, and how do you then proceed to rearrange the labels?
Note: (1/19/2003 1:23AM) Edited to add that the boxes are opaque.
|
| STUPID |
What is the beginning of eternity, the end of time and space, the start of every end, and the end of every race?
|
| CLIMBING SNAIL |
A snail is at the bottom of a well that is 20 meters in depth. Every day the snail climbs 5 meters upwards, but at night it slides 4 meters back downwards. How many days must elapse till the snail reaches the top of the well?
|
| 8-WAY CAKE SLICE |
You have a round birthday cake. With three straight slices of a knife, divide the cake into 8 equal pieces. I know of two different solutions.
|
| CHESSBOARD SQUARE COUNT |
How many squares are on a chessboard (8 x 8)?
Followup 11/24/2002 7:44PM: How many rectangles are on a chessboard?
|
| MONTY HALL SHOW |
You are a contestant on the Monty Hall game show. Three closed doors are shown before you. Behind one of these doors is a car; behind the other two are goats. The contestant does not know where the car is, but Monty Hall does.
The contestant picks a door and Monty opens one of the remaining doors, one he knows doesn't hide the car. If the contestant has already chosen the correct door, Monty is equally likely to open either of the two remaining doors.
After Monty has shown a goat behind the door that he opens, the contestant is always given the option to switch doors. What is the probability of winning the car if she stays with her first choice? What if she decides to switch?
Hint: Like many other problems on this site, the first answer that comes to mind tends to be wrong. Try enumerating the possible outcomes in a tree-like structure, recording the probabilities of each event along the way.
Note: This riddle was popularized by Marilyn vos Savant, current holder of the world's highest IQ. She introduced it in a magazine puzzle column, and was subsequently bombarded by flame mail accusing her of having the wrong solution, even though she was right. Even statistics professors were fooled! Today, this riddle is mentioned in almost every probability class.
|
| GLASS HALF FULL |
You are in an empty room and you have a transparent glass of water. The glass is a right cylinder, and it looks like it's half full, but you're not sure. How can you accurately figure out whether the glass is half full, more than half full, or less than half full? You have no rulers or writing utensils.
Hint 1: To help you get started if you're stuck, here's a solution that's not good enough. Holding the cup upright, use the palm of your left hand to cover the cup's opening. Now make a pinching gesture with the index finger and thumb of your right hand. Put the thumb at the base of the cup, and the index finger adjacent to the water level, thereby gauging the height of the water surface from the base of the cup. Now freeze the distance between those two fingers. Flip the cup upside down with your left hand; no water falls out since you've sealed the opening with your left palm. Now put your frozen right hand against the cup, and see if the inverted water level is next to your index finger. If so, the cup is exactly half full. This seems like a good solution, but it's actually slightly inaccurate, because the palm of your hand is not a perfectly flat surface. Also, you'll most likely lose some water when you flip the cup upside down. We want a really accurate method.
Hint 2: Utilize the geometry of the cup. That's really important.
Hint 3: Don't drink the water. ^_^
Note: My friend David Lau found this riddle in a book designed for little kids. Hopefully you can solve it :)
|
| HANGING CHAIN |
You have a 6-foot long chain that is suspended at its ends, tacked to a wall. The tacks are parallel to the floor. Due to gravity, the middle part of the chain hangs down a little bit, forming a hump; the length of this hump in the vertical direction is 3 feet. Find the distance in between the tacks.
Note: asked at m$ interview.
|
| TWO CONDOMS, THREE WOMEN |
Willywutang would like to have safe sex with three women, any of whom may be carrying an STD. Given two condoms, how can he do so, while ensuring that no STD is passed from one woman (or possibly himself) to another (or to himself)?
|
PRIME PAIRS
M |
Pairs of primes separated by a single number are called prime pairs. Examples are 17 and
19. Prove that the number between a prime pair is always divisible by 6 (assuming both
numbers in the pair are greater than 6).
|
| MYSTERIOUS TRIANGLE AREA |
The second triangle is formed by rearranging pieces used to create the first. Yet there is a strange gap in the second triangle. Has area vanished? Is the conservation of matter bogus? Explain this madness.
Hint: "Believe nothing, no matter where you read it, or who said it, no matter if I have said it, unless it agrees with your own reason and your common sense." - Buddha
|
| MYSTERY MATRIX I |
5 3 7 8 9
2 6 4 1 x
8 0 2 0 4
What is x?
|
| haha |
What gets wetter the more it dries?
|
8 QUEENS
C |
Place 8 queens on a chess board in such a way that they cannot capture each other.
Note: asked at m$ interview.
|
| KNIGHT VS. DRAGON |
A dragon and knight live on an island. This island has seven poisoned wells, numbered 1 to 7. If you drink from a well, you can only save yourself by drinking from a higher numbered well. Well 7 is located at the top of a high mountain, so only the dragon can reach it.
One day they decide that the island isn't big enough for the two of them, and they have a duel. Each of them brings a glass of water to the duel, they exchange glasses, and drink. After the duel, the knight lives and the dragon dies.
Why did the knight live? Why did the dragon die?
Note: From a Trilogy interview.
|
| COIN UNBIASING |
You and your arch rival are competing for the same girl. After years of battling, you both decide to settle it by tossing a coin.
Your rival produces a coin, but you don't happen to have one on you. You are certain that the coin your rival has produced is loaded, ie. it will come up with heads more than 50% of the time on average.
How do you arrange a fair contest, based purely on chance and not skill, by flipping this coin?
Variation: (COIN BIASING) You and your rival are competing for the same girl, and decide to settle it with a coin toss. Your rival has known the girl longer than you have, so you agree that it is fair for him to have a chance of winning equal to P, where P > 0.5. However, you only have a fair coin. How can you conduct this contest such that the biased probability is manifested? What is the average number of coin flips needed to determine a winner?
|
| WHO AM I? (1) |
I am greater than God, and more evil than the devil. Poor people have me. Rich people want me. And if you eat me, you'll die. What am I?
|
| PLACING TREES |
You are a landscape specialist, and have been asked to design a garden for a math professor. He wants four trees that are all equidistant from each other. How do you place the trees?
|
| ANCHOR |
A boat of mass M1 is floating in a lake of water. The volume of the lake is V. The water surface is initially at height h, as measured relative to the lake's floor. There is an anchor of mass M2 sitting on the boat's deck. A person standing on deck picks up the anchor and throws it overboard. The anchor then sinks to the bottom of the lake, and the water surface height becomes h'.
Which of the following qualitiative relationships is correct? What assumptions are you making about the values of M1, M2, h, and V?
Note: From the US Navy's nuclear power program interview for naval officers!
|
| NEW DOOR SCRAMBLE |
Can you rearrange the letters of new door to make one word?
|
| MAGNET DETECTION |
You have two cylindrical rods of iron, identical in size and shape. One is a permanent magnet. The other is just non-magnetized iron -- attractable by magnets, but not permanently magnetic itself. Without any instrument, how can you determine which is which?
|
| 27 CUBES |
A cube is to be cut into 27 smaller cubes (just like a Rubik's Cube). It is clear that this can be done with 6 cuts to the original cube (2 in the x, 2 in the y, 2 in the z). Now, assuming that you can arrange the pieces however you like before doing a cut, what is the minimum number of cuts required to obtain the 27 smaller cubes? Prove your answer.
|
| ICE CREAM URBAN RIOTS |
Scientific studies have discovered a direct, positive correlation between eating ice cream and the occurrence of massive urban riots. Why?
|
| CHAIN LINK 21 |
What is the least number of links you can cut in a chain of 21 links to be able to give someone all possible number of links up to 21?
|
| HANGING VS. BEHEADING |
A guy is sitting in some foreign country in death row awaiting his execution the next day. The executioner decides to grant him one last favor; he'll give him a choice in the execution method. The prisoner is therefore allowed to make one last statement. If this statement is true, he'll be hanged the next day. If however his statement is false he will be beheaded the next day. What should the prisoner say?
|
| 9 DOTS |
You have 9 dots arranged like a rectangle:
. . .
. . .
. . .
Without lifting your pen, draw four lines that cross all 9 dots.
Good Hint: This is a very famous problem. It was actually responsible for the cliche: "Think outside the box."
|
| 222 |
Use the homophones "to", "too", and "two" in one question.
|
| ALL HORSES SAME COLOR |
Do you agree with the following inductive proof? Clearly explain why or why not.
Theorem: All horses are the same color.
Base Case: 1 horse. Clearly with just 1 horse, all horses have the same color.
Inductive Step: If it is true for any group of N horses that all have the same color, then it is true for any group of N+1 horses. Given any set of N+1 horses, if you exclude a random horse, you get a set of N horses. By the inductive step these N horses all have the same color. But by excluding any other horse in the pack of N+1 horses, you can conclude that the last N horses also have the same color. Therefore all N+1 horses have the same color. QED.
|
| BALLOON IN CAR |
You have a car with a very flat roof, on a level road. There's a helium
balloon in the car, barely scraping the roof - any slight force will move
it. You start the car and accelerate forward very fast. Does the balloon move
with respect to the car? If so, how? (This does not depend on wind from open
windows or anything tricky.)
|
| DESPERADOS IN JAIL |
A Sheriff has captured a gang of ten desperados. His jail has only nine
cells, and he cannot put more than one man into any one cell. What
should he do? He tries taking the first two men and putting them into
the first cell. The third deperado is put into the second cell, the
fourth into the third, and the fifth into the fourth. The sixth,
seventh, eigth and ninth men go into cells five, six, seven and eight
respectively. Then then goes back to the first cell, where he originally
put two men, and move the last man from there into cell nine. Has he
solved the problem? Why or why not?
|
| MARBLE JARS II |
A jar contains one hundred marbles, each of which may be white or black. You pull out 100 marbles with replacement, and they are all white. What is the probability that all one hundred marbles are white?
Note: "With replacement" means you take out a random marble, look at its color, then put that marble back. Then repeat.
|
| HOW MANY STARS IN THE UNIVERSE? |
I’m going to ask you if there are more than 6.02 x 10^23 stars in the universe. Write the answer on a piece of paper. Make sure that everyone will agree you have written the correct answer on the paper.
|
| EVIL MAYOR |
In a certain town lived a miller, his daughter, and the evil mayor. The miller was in debt to the mayor, and the mayor had his eye on the miller’s daughter. The mayor made a proposition: he would place a black stone and a white stone in a bag, and the miller’s daughter would pick one out in front of the whole village. If she drew the white stone, the mayor would forgive the miller’s debt. If she drew the black stone, the mayor would marry the miller’s daughter and take the mill. The miller had no choice but to agree. The miller’s daughter has no reason to trust the mayor, and believes that he will place two black stones in the bag. How can she get out of marrying the mayor and save the mill?
|
| FEMINIST RULE |
The overly feminist rulers of a country decide that
there are too many baby boys being born. The rulers
decide to enforce a new law concerning child birth on
their overly prosperous subjects. Each family is
permitted to have as many children as they want,
provided that they only produce baby girls. Once a baby
boy enters the family, the family is no longer
permitted to have children. Assuming each law abiding
family wants to have as many children as possible, what
will happen to the ratio of boys to girls, and why?
- the ratio of boys to girls will go up.
- the ratio of boys to girls will stay the same.
- the ratio of boys to girls will go down.
|
| LETTER SEQUENCE |
Click here to listen to the problem statement.
Now highlight the area below with your mouse to see the partial sequence:
W I T N L I T _
|
| DICE CORNER |
You're looking through a hole, at the corner of a regular, normal die. The below image shows all that you can see. Can you identify AT LEAST ONE of the sides visible through the hole?
|
| TRAIN BRIDGE |
A man is 3/8's of the way across a train bridge, when he hears the whistle of an approaching train behind him. It turns out that he can run in either direction and just barely make it off the bridge before getting hit. If he is running at 15 mph, how fast is the train traveling? Assume the train travels at a constant speed, despite
seeing you on the tracks.
Note: From a 7th grade pre-algebra book.
|
| MYSTERY MATRIX II |
What goes in the blank?
_ T T F F
S S E N T
E T T F F
S S E N T
Hint: it's not 'E'
|
| POISON DRINKS |
A rich old man has died. After his death, his children are surprised to learn that he has left all of his money to his oldest son Jeremiah, who loved him dearly, and ignored his other children, who hated him.
So, the funeral is a day or two later, and the other sons and daughters have decided to kill Jeremiah and take his inheritance. Since his father's death, Jeremiah has taken to drinking, and they know that, at the wake, he's going to be gulping down the liquor like it was nectar of the gods. So they decide to poison the drinks. One of the other sons, Wallace, tends bar, and gets the poison all ready.
So Jeremiah comes up, crying and depressed, and orders a scotch on the rocks. Wallace serves him one, and he chugs it down in two seconds. "Give me another." Wallace gives him a second glass of scotch, which he also drinks in a matter of moments. The other siblings are puzzled...the poison is fast-acting; Jeremiah should be convulsing on the floor and retching his guts out. Finally, fifteen minutes later, a rather inebriated and very much alive Jeremiah orders one last glass of scotch, but as Wallace hands it to him, he changes his mind and leaves, sobbing. The other siblings come over to Wallace, and wonder what's going on. They talk about what could have gone wrong for a few minutes, and figure the poison's harmless. So Wallace sips the drink he poured for Jeremiah, and is pronounced DOA thirty minutes later.
Why did Jeremiah live? (He had no immunity to the poison, he didn't know it was coming, and the poison was obviously deadly.)
|
| ANT ON A BOX |
A 12 by 25 by 36 inch box is lying on the floor on one of its 25 by 36 inch faces. An ant, located at one of the bottom corners of the box, must crawl along the outside of the box to reach the opposite bottom corner. It can walk on any of the box faces except for the bottom face, which is in flush contact with the floor. What is the length of the shortest such path?
|
| 100 M RACE |
Sam and Max run a 100 meter race. Sam wins by five yards. To make it sporting, he starts 5 yards beind the original start line in the second race. Assuming both runners run at the same speed, who wins the second race? The challenge is to solve this problem without doing any algebra.
|
| TWO GIRLFRIEND PARADOX |
Bill has two girlfriends, Hillary and Monica. Monica lives in the East of a city, and Hillary lives in the West of the same city, as shown in the figure below. Once every morning at a random time, Bill arrives at the train station at the center of the city. A train leaves for the East every 10 minutes, and a train leaves for the West every 10 minutes — Bill chooses whichever train arrives first. On average, could Bill end up with one girl more often than the other? If so, how many times more often? Why?
|
| SMARTER THAN MENSA |
Mathematicians normally disparage ambiguity and sensitize themselves to its symptoms, so as to detect and correct it, more than do many other intellectuals. For example, intelligence tests used by American MENSA, a self-styled “American High I.Q. Society”, are notorious for unintended ambiguities that elicit “incorrect” responses from more imaginative and intelligent test takers, thus thwarting the tests’ ostensible purposes. It's rather ironic. The following questions, framed by a MENSA psychologist, came from a box of Raisin Bran ©. For each question devise as many answers as you can, all at least as valid as the one answer the psychologist deemed “correct”.
- Which of the following five words doesn't belong with the others, and why?
| pail |
skillet |
knife |
suitcase |
card |
- One of the figures below lacks a characteristic common to the other figures. Which one, and why?
- One of the figures below lacks a characteristic common to the other figures. Which one, and why?
|
| GRAMMAR DISTINCTIONS |
English grammar used to be taught as an analytical subject, but today such rigorous treatment is rarely seen in the States. Consequently, most modernized Americans are unable to discern the differences between the following four sentences:
- Only birds read poetry.
- Birds only read poetry.
- Birds only read poetry. (two different interpretations for this sentence exist)
- Birds read only poetry.
For each of these sentences, write a sentence or two showing that you appreciate the distinctions.
Bonus Question: Translate each of the above sentences into formalized logical expressions, using boolean logic symbols and quantifiers (e.g. and, or, not, implies, for all, there exists, etc).
|
| MYSTERY MATRIX III |
E, O,
E, R,
E, ?,
N, ?,
E, N
What goes in the question marks?
|
| CONFUSED BANK TELLER |
A confused bank teller transposed the dollars and cents when he cashed a check for Ms Smith, giving her dollars instead of cents and cents instead of dollars. After buying a newspaper for 50 cents, Ms Smith noticed that she had left exactly three times as much as the original check. What was the amount of the check?
|
| TWO LADDERS |
Two ladders are placed cross-wise in an alley to form a lopsided X-shape. Both walls of the alley are perpendicular to the ground. The top of the longer ladder touches the alley wall 5 feet higher than the top of the shorter ladder touches the opposite wall, which in turn is 4 feet higher than the intersection of the two ladders. How high above the ground is that intersection?
|
| SPY SATELLITES |
The NSA has a large number of spy satellites in geosynchronous orbit; if I told you the exact number, I'd have to kill you. These satellites communicate continuously by microwaves with stations on the Earth, and with each other -- except when the Earth’s bulk interrupts the line-of-sight path that microwaves need. Prove that at all times, at least two satellites are each in uninterrupted communication with the same number of satellites.
|
| COLLECTOR VIOLINS |
Violins produced on the island of Grxcd have become collectors’ items since it sank into the
sea two centuries ago. All the island’s violins were produced by Bropcs or one of his sons, or by
Czwyz or one of his sons. Every violin was labelled ostensibly to reveal its maker but, although
Bropcs and his sons always labelled their violins truthfully, Czwyz and his sons always labelled
their violins with falsehoods. Both families playfully interfered with collectors’ attempts to
establish provenances for their violins. For example, collectors figured out that a violin labelled
“ This violin was not made by any son of Bropcs.” was made by Bropcs Sr.; can you see why?
The most desirable violins are so labelled that a connoisseur can tell that it must have been made
by one of the fathers, either Bropcs Sr. or Czwyz Sr., but cannot tell which. How might such a
violin be labelled?
|
| OPEN POKER |
In this variation on the game of Poker, two people play as follows: Player 1 takes any 5 cards of his choice from the deck of 52 cards. Then player 2 does the same out of the remaining 47. Then player 1 may choose to discard any of his cards and replace them from the remaining 42. Then player 2 may discard any of his cards and replace them, but he may not take player 1's discards. ALL of the transactions with the deck are public knowledge, unlike the real game of Poker.
After this process, the winner is the one who has the better poker hand. For the benefit of those who have not played poker, these are the highest ranking hands, in decreasing order of value:
- Royal Flush: the A K Q J 10 of the same suit.
- Straight Flush: any five consecutive of one suit. Highest card of the five is the tiebreaker. No one suit is more powerful than another.
- Four of a kind: all four of one rank (i.e. four aces). A hand with 4 aces outranks 4 kings, etc.
- Full house: a pair of one rank and 3-of-a-kind in another rank, i.e. Q Q 8 8 8.
- Flush: Any 5 cards of the same suit that don't satisfy #2.
Because of the clear advantage of player 1, the win is given to player 2 if the hands are equal in strength.
Which player would you rather be? What strategy do you use?
|
| MAKING A DOLLAR |
You are given n coins of denominations 1, 0.5, 0.25, 0.1, 0.05 and 0.01 (6n coins altogether). You are then asked to choose n out of these 6n coins that sum up to exactly 1. What is the smallest n for which this is impossible?
|
| ALL SIGS ARE FALSE |
A certain UberPuzzler in a certain puzzle forum uses the signature "All signatures are false". What is the most that can be deduced from this statement alone (i.e. without any knowledge of other signatures)?
( with apologies to J.F. )
Note: If you liked this recursive statement, you might also like "Why is there no correct answer to this question?" and "A man comes up to you and says 'I am lying.' Can you conclude anything?"
|
| THE CLEVER GRANDFATHER |
A stopped clock gives the exact time twice a day, while a normally running (but out of sync) clock will not be right more than once over a period of months. A clever grandfather [as in grandfather clock] adjusted his clock to give the correct time at least twice a day, while running at the normal rate. Assuming he was not able to set it perfectly, how did he do it?
|
| GEOGRAPHY TEST |
On a geography test you have to tell which of two German cities is greater in population for all possible pairs of the 80 largest cities of Germany. (And that's the only task on the test since it's already 5 pages long.) But you didn't study last night, and only even recognize half the cities, and don't even know how those are ordered relative to each other. Your friend on the other hand studied dutifully all night and recognizes all the cities and even knows how two cities are ranked relative to each other 60% of the time.
A week later you get the test-result and you have a higher score than your friend. How come?
|
LOG MOTOR
P |
There's a perfectly cylindrical log mounted horizontally on frictionless pins at each end. It is in a container, set up so that, looking down the length of the log, on one side is air and the other is water. There are walls to keep the air and water separated, and these walls meet the log lengthwise with frictionless seals. Given that a log floats in water, would the log start spinning? Why or why not? See diagram for cross-sectional view.
Note: Originally an interview question for a mechanical engineering position!
|
| TRUE OR FALSE LIST |
Consider a list of 2000 statements:
1) Exactly one statement on this list is false.
2) Exactly two statements on this list are false.
3) Exactly three statements on this list are false.
. . .
2000) Exactly 2000 statements on this list are false.
Which statements are true and which are false?
What happens if you replace "exactly" with "at least"?
What happens if you replace "exactly" with "at most"?
What happens in all three cases if you replace "false" with "true"?
Note: "The 'exactly . . . false' problem was posed by David L. Silverman for 1969 statements in the January, 1969 issue of the Journal of Recreational Mathematics. I got the problem from Martin Gardner's "Knotted Doughnuts and Other Mathematical Entertainments", where he discusses it and some of the variants above." - Paul Sinclair
|
| MONEY IN BOOK |
There is a rich man living with two other people his butler and his maid. One day the rich man is sitting at his desk counting his money, preparing to deposit it at the bank. When he is done he goes to the bank, but when he arrives, he realizes he left a $100 dollar bill on the desk. So he quickly called the house and told the butler that he forgot the $100 bill on the desk, and he will come home now to pick it up. When he arrived home he asked the butler what he did with the bill, the butler said he put it under the green book on the desk. When the rich man looked under the book, it wasn't there, so he asked the maid if she saw it. She said she saw the $100 bill when she was dusting and put it between pages 67 and 68 of the green book. Right then and there the rich man called the police, and knew who stole it, even before the rich man checked inside the book to see if the $100 bill was there. How did he know?
Note: Technically the book must have a certain property for this trick to work, but you would be hard pressed to find a book without this property.
|
| CENTURY FINAL DAY |
Assuming year 1 started on Sunday, it can be shown that only some days are possible as a century's final day. What are these days?
|
| DOG, CHICKEN, RICE |
(Classic puzzle) A farmer returning home from the market must get across the river and return home with his three purchases, a dog, a chicken and a bag of rice. However, He must take them in his boat. He can't have more than one item with him on his boat at all times. He cannot leave the dog alone with the chicken because the dog will eat the chicken, and he cannot leave the chicken alone with the bag of grain because the chicken will eat the bag of grain. How does he get all three of his purchases back home safely?
Note: (1/19/2003 12:59AM) Corrected a wording error.
|
| WINDING VINE LENGTH |
There is a tree 20 feet high, with a circumference of 3 feet. A vine starts at the base of the tree and winds around the tree 7 times before reaching the top. How long is the vine?
Hint: There is an easy way to solve this problem which only uses junior high school math!
Note 1: Apparently from Chinese texts over 2000 years old.
Note 2: Treat the tree as a perfect cylinder.
|
| THREE PRIMATES |
You walk into a room in which there are three primates: a chimpanzee, an orangutan, and a gorilla. The chimpanzee is holding a banana in each hand, the orangutan is holding a big stick, and the gorilla is holding nothing. Which primate in the room is the smartest?
|
| HOW MANY LETTERS IN CORRECT ANSWER |
How many letters does the correct answer to this puzzle contain?
|
| TAKING PILLS |
A patient has fallen very ill and has been advised to take exactly one pill of medicine X and exactly one pill of medicine Y each day, lest he die from either illness or overdosage. These pills must be taken together. The patient has bottles of X pills and Y pills. He puts one of the X pills in his hand. Then while tilting the bottle of Y pills, two Y pills accidentally fall out. Now there are three pills in his hand. Because both types of pill look identical, he cannot tell which two pills are type Y and which is type X. Since the pills are extremely expensive, the patient does not wish to throw away the ones in his hand. How can he save the pills in his hand and still maintain a proper daily dosage?
|
| JAPANESE KINDERGARTEN APPLICATION |
Given the following information, what is 10 + 10?
1+1=0; 2+2=0; 3+3=0; 4+4=2; 5+5=0; 6+6=2; 7+7=0; 8+8=4; 9+9=2; 10+10=?
Note: Supposedly this riddle is from an application to a Japanese kindergarten! Amusingly I didn't get the correct solution myself, so I guess my academic career would've been toasted real early if I lived in Japan. God Bless America! =D
|
| MATCH PUZZLES |
A bunch of nifty match configuration problems from forum regular [BNC]. Starting with the following configuration:
- -
| | |
- -
| | |
- -
- Remove two matches to get two squares -- one larger than the other.
- Move 3 matched to get 3 identical squares.
- Move 4 matched to get 3 identical squares.
- Move 2 matches to get 7 (non-identical) squares.
hint: you may place one match over another
- Move 4 matches to get 10 (non-identical) squares.
- Move 8 matches to get 6 identical squares.
|
| OFF-CENTER WHEEL |
2/4/2003 2:55AM
Willywutang looked at the piece of wood before him. It is a 10” diameter round, flat piece he wanted to use as a “wheel” in his art lesson homework. Alas! While drilling the center hole (1” diameter) , he sneezed, and the hole is way off-center (although, luckily, still within the “wheel” – the center of the hole is 3” away from the center of the wheel).
And then, revelation! Willy cuts the wheel into two parts, glued them again, and got a perfectly centered hole in the wheel. How did he do it?
Note: assume Willy has in his possession a magical cutter that cuts with zero width, and magic glue that can glue pieces with zero distance between them.
|
| MATCH EQUATIONS |
2/4/2003 2:55AM
In each of the following three configurations of matches, move a single match to form valid equations. The = and + are composed of two matches each.
- \/|+|=\/
- \/|+|=\/|
- \/|+|=|||
|
| UNUSUAL PARAGRAPH |
2/4/2003 2:55AM
How quickly can you find out what is so unusual about this paragraph? It looks so ordinary that you would think that nothing is wrong with it at all, and, in fact, nothing is. But it is unusual. Why? If you look at it, study it and think about it, you may find out, but I am not going to assist you in any way. You must do it without coaching. No doubt, if you work at it for long, it will dawn on you. Who knows? Go to work and try your skill. Par is about half an hour. So jump to it and try your skill at figuring it out.
Good luck --don't blow your cool.
Hint 1: I thought long and hard about this. "RJ, " I said, "a solution is probably right in front of you. Just chill out and it will just pop into mind." I sat and thought and sat and thought but I'm still waiting. I'm probably not as smart as I think I am. - R. Jacobus
Hint 2: RJ, don't quit. You'll find that paragraph's odd quality, but you must stay with it. My old typing instructor taught my class this quizzical brain-twisting oddity many moons ago, so I had a solution right away, but I know you can do it if you studiously put your mind to it. - Wolfgang
|
| TWO CONSECUTIVE HEADS |
2/4/2003 2:55AM
An unbiased coin is tossed n times. What is the probability that no two consecutive heads appear?
|
| HOTEL INFINITY |
3/9/2003 4:08AM
So, an eccentric entrepreneur by the name of Alphonse Null has sent out a press release about his new, mind-blowing hotel: The Hotel Infinity. Null informs the world that this hotel has an infinite number of rooms (specifically, an infinity equal to the cardinality of the integers). A quick tour puts skeptics' claims to rest; as far as anyone can tell, this hotel has infinite rooms. The consequences are mind-boggling, and Null sets up a press conference to answer questions...
"So, Mr. Null, how will patrons get to their room, if their room number has, say, more digits than protons in the universe?"
"The elevators have an ingenious formula device instead of buttons... simply input the formula for your room number, with Ackermann numbers or somesuch... your room formula can be picked up at the front desk. There's not even any need to know what the formula means!"
"How do you produce the power and water for this hotel?"
"I have infinite generators and wells, of course. This IS an infinite hotel, you know! *chuckle*"
"What about costs? How much will it cost to stay here?"
"That's the beauty of it! Since there are as many positive even integers as there are integers, I can change the same price to only every other room and still make the same profit! I could charge only every millionth room... each guest has a one-in-a-million chance of not getting a free room, and I still get paid the same! I love the properties of infinite sets, especially when it comes to profit!"
"But, Mr. Null... I think you've made a severe mistake in your assumptions regarding profit..."
"Oh?"
The reporter then mentioned something which made Mr. Null's face turn white.
"Oh... oh goodness... THIS PRESS CONFERENCE IS OVER!" Then he ran out.
Assuming that everything Null said about the hotel is true: it really is infinite; it really is easy to get to your room; it really can generate infinite power for the guests; the cardinality of the set of multiples of a million, is the same as the cardinality of the integers...
So with what simple assumption did Mr. Null go wrong?
Note: "(There's one assumption I'm looking for, although any other assumption which would work is fine too.)" - Jeremiah Smith, writer of this puzzle
|
| TOO MANY SOLUTIONS |
3/9/2003 4:11AM
It is known that a quadratic equation has either 0, 1, or 2 unique real solutions. Well, look at this equation:
Without loss of generality, assume a < b < c. Now note that x=a, x=b, and x=c are all unique solutions! How can this equation have 3 solutions?!
|
| ESP PUZZLES |
4/7/2003 12:17AM
The many incarnations of these simple online "mind-reading" magic tricks have stupefied many a surfer. Hopefully you will not be duped too?
- Mind-Reading Card Selection: http://sprott.physics.wisc.edu/pickover/esp.html
- Mind-Reading Symbol Selection: http://mr-31238.mr.valuehost.co.uk/assets/Flash/psychic.swf
Note: Try to rigorously explain what makes the second trick work.
|
| TWO IDENTICAL SPHERES |
4/7/2003 12:17AM
You are given 2 identical looking spheres. They have the same mass and have the same diameter. Physically, they look the same, and have the same surface texture. (ie you can't visually pick them apart) They are both hard, thus they won't bounce and they won't have any 'give'. They both have perfectly smooth surface.
One is made of less dense material and is soild and uniform through out. The other is made of higher density material, but since having the same mass and volume as the other, it is hollow at its centre (assume a spherical cavity with the centre of cavity and centre of the whole sphere at the same point).
With a minimum of instruments, how can you determine which one is hollow and which one is solid?
Note: (4/7/2003 12:36AM) Many of my friends here at UC Berkeley have been asked this question at tech interviews recently (Microsoft, Amazon.com, etc.)
|
| FRYING FOOD |
4/7/2003 12:17AM
The boiling point of olive oil is higher than the melting point of tin. If Italian skillets are made of tinned copper, how can they be used to fry food in olive oil?
Note: Originally posed to Enrico Fermi by one of his students.
|
21 FACTORIAL
M |
4/7/2003 12:17AM
21!=510909x21y1709440000
Without calculating 21!, what are the digits marked x and y?
|
| BICYCLE TUGGING |
5/11/2003 10:47PM
Consider a bicycle as shown in the picture below. It is perfectly normal except for a piece of string caught in the rear wheel. If we pull the string in the direction P, will the bicycle move forward, move backward, or 'stay put'? Assume that the wheel does not slip on the ground.
|
| SUM OF REAL NUMBERS |
5/11/2003 10:59PM
The sum of N real numbers (not necessarily unique) is 20. The sum of the 3 smallest of these numbers is 5. The sum of the 3 largest is 7. What is N?
|
| RIVER CROSSING |
5/11/2003 10:59PM
Two people come to a river. There is a boat, however it can carry one person only. How can they each get to the other side of the river using the boat?
Note: The problem is too open-ended to have only one solution, but think of the most elegant possible scenario.
|
| TWIDDLING BOLTS |
Contributor: William Wu
|
5/11/2003 10:59PM
|
The bolts shown above have regular helical grooves. If you circle the bolts around each other in the directions indicated, in the way you would twiddle your thumbs, will the bolt heads:
- move away from each other,
- move toward each other, or
- stay the same distance apart?
Explain your reasoning. Note that you do not rotate either bolt around its own axis, and you always keep the bolts closely in contact with one another.
|
| DOMINANT FIFTH |
Contributor: Nootch
|
9:00 PM 8/19/2004
|
"What's the Dominant Fifth?" asked Dr. Dingo, as his daughter Cicely came in from school.
Cicely blushed. "Just a secret society," she said. "I'm one of the vice-presidents."
"And you're meeting tonight; is that right?"
"How on earth did you know?" ask Cicely.
"You left this lying about. That's no way to keep secrets, my girl." He handed Cicely this paper:
Dominant Fifth
REASM NCNVE OTMLE SEHST TAOEI
"How did you manage to read it?" asked Cicely. "The code is known to only about eight of us."
"Change it," said Dingo. "Any fool can read that."
Where and When is the next meeting scheduled?
|
| CUTE PERMUTATION INEQUALITY |
Contributor: William Wu
|
9:00 PM 8/19/2004
|
You are given a finite sequence of n real values (a1,...,an). Prove that for any permutation  of {1,...,n}, of {1,...,n},
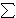 i=1 to n ai a i=1 to n ai a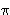 (i) <= (i) <= 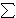 i=1 to n ai2 i=1 to n ai2
At least one two-liner quickie proof exists.
|