| relatively medium |
| GLOBE TRAVERSAL |
how many places are there on the earth that one could walk one mile south, then one mile
west, then one mile north and end up in the same spot? to be precise, let's assume the
earth is a solid smooth sphere, so oceans and mountains and other such things do not
exist. you can start at any point on the sphere. also,
the rotation of the earth has nothing to do with the solution; you can assume you're walking
on a static sphere if that makes the problem less complicated to you.
Hint 1: think you've figured it out? do you know that there's more than one? in fact, there
are more than two. also note that walking north from the north pole (or south from
the south pole) is illogical and therefore does not enter into the problem. all normal
assumptions about directions will be used.
Hint 2: christopher columbus.
|
| GHETTO ENCRYPTION I |
You want to send a valuable object to a friend securely. You have a box which can be
fitted with multiple locks, and you have several locks and their corresponding keys.
However, your friend does not have any keys to your locks, and if you send a key in an
unlocked box, the key could be copied en route. How can you send the object securely?
Alternative, more precise phrasing: Andy and Grant are staying in different rooms in the same hotel. Andy needs to give a gold pendant to Grant, but spies are trying to assassinate Andy and Grant so neither of them can leave their room. The only way they can transfer objects is by using the bellhops. Both Andy and Grant have a safe with a large clasp that can be secured with a padlock. Both Andy and Grant have a padlock and a corresponding key. (So 1 gold pendant, 2 safes, 2 padlocks, and 2 keys.) But the bellhops are thieves. Anything that is not padlocked in the safe will be stolen by the bellhops - including any unlocked padlocks, the keys or the pendant. How can Andy transfer the gold pendant to Grant without it being stolen? (where both sides have encryption capability, and where unsecured items are taken away rather than just copied?)
|
| GHETTO ENCRYPTION II |
Three coworkers would like to know their average salary. However, they are self-conscious
and don't want to tell each other their own salaries, for fear of either being ridiculed
or getting their houses robbed. How can they find their average salary, without disclosing
their own salaries?
|
| ADJACENCY GRID |
arrange the numbers 1 to 8 in the grid below such that adjacent numbers are not in adjacent boxes (horizontally, vertically, or diagonally).
the arrangement above, for example, is wrong because 3 and 4, 4 and 5, 6 and 7, and 7 and 8 are adjacent.
|
| FAUSTIAN ROUND TABLE COIN GAME |
you die and the devil says he'll let you go to heaven if you beat him in a game. the
devil sits you down at a round table. he gives himself and you a huge pile of quarters.
he says "ok, we'll take turns putting quarters down, no overlapping allowed, and the
quarters must rest on the table surface. the first guy who can't put a quarter down
loses." you guys are about to start playing, and the devil says that he'll go first.
however, at this point you immediately interject, and ask if you can go first
instead. you make this interjection because you are very smart, and you know that if you
go first, you can guarantee victory. explain how you can guarantee victory.
|
| DOMINOES ON A CHESSBOARD |
using 31 dominoes, where one domino covers exactly two squares, can you cover
all the empty squares on this chessboard (which has only 62 spaces, since two opposite corner squares are removed). if so, how? if not, why? prove your claim.
|
| MANHOLES |
Why are manholes round?
Note: This is a famous Microsoft question. Yet amusingly, the Microsoft campus uses square manholes.
|
| SQUARE DIVISION |
draw a square. divide it into four identical squares. remove the bottom left hand square. now divide the resulting shape into four identical shapes.
|
| EQUILATERAL TRIANGLE DIVISION |
draw an equilateral triangle (all sides same length). divide it into four identical shapes. remove the bottom left hand shape. now divide the resulting shape into four identical shapes.
|
| LIGHT BULBS AND SWITCHES |
You are in a room with three light switches, each of which controls one of three light bulbs in the next room. Your task is to determine which switch controls which bulb. All lights are initially off, and you can't see into one room from the other. You are allowed only one chance to enter the room with the light bulbs. How can you determine which lightswitch goes with which light bulb?
|
| PUNCTUATION I |
Add punctuation to the following phrase to make something gramatically and logically coherent:
is is not not not is not is is is is not is not is it not
|
| PAPER CUTTING |
You have a 5x5 piece of paper. Two diagonally opposite corners of this paper are truncated as shown in the diagram below. You also have scissors. Show how to cut up the 5x5 paper into two pieces, so that the two pieces can then be interlocked to form a 6x4 rectangle.
|
| POPULATION OF FUNKYTOWN |
In the city of Funkytown, the following facts are true:
- No two inhabitants have exactly the same number of hairs.
- No inhabitant has exactly 483,207 hairs.
- There are more inhabitants than there are hairs on the head of any one inhabitant.
What is the largest possible number of inhabitants of Funkytown?
Note: I recently (11/24/2002 8:00PM) read this puzzle in a book by Henry Ernest Dudeney, England's greatest puzzle creator. So writing credits to him.
|
| CHOCOLATE MILK |
You have two thermoses. The first contains a liter of milk, the second contains a liter of pure chocolate syrup. You pour one cup of milk out from the first thermos to the second one. Then, after mixing that, you take one cup of the mixture from the second thermos, and pour it back into the first thermos. After completing these two operations, which thermos is more pure?
|
| BROWN EYES AND RED EYES |
There is an island of monks where everyone has either brown eyes or red eyes. Monks who have red eyes are cursed, and are supposed to commit suicide at midnight. However, no one ever talks about what color eyes they have, because the monks have a vow of silence. Also, there are no reflective surfaces on the whole island. Thus, no one knows their own eye color; they can only see the eye colors of other people, and not say anything about them. Life goes on, with brown-eyed monks and red-eyed monks living happily together in peace, and no one ever committing suicide. Then one day a tourist visits the island monastery, and, not knowing that he's not supposed to talk about eyes, he states the observation "At least one of you has red eyes." Having acquired this new information, something dramatic happens among the monks. What happens?
Hint: First consider the case where there are only a few monks on the island, some with brown and some with red. Work through the logic and find out what happens over time. Then generalize for the case of M monks on the island, N of which have red eyes.
Update 10/15/2002 12:14AM: What happens if we change the tourist's statement to each of the following?
- "There are 10 Brown Eyed Monks"
- "There are at lesat two Red Eyed Monks"
- "There is an odd number of Red Eyed Monks"
- "There is an even number of Red Eyed Monks"
- "There is more than one Red Eyed Monk"
|
| WHERE'S THE FATHER? |
The mother is 21 years older than the child. In 6 years from now, the mother will be 5 times as old as the child. Question: Where's the father?
|
| .999 ... |
Compare the numbers 0.99999... (infinitely many 9s) and 1. Which of the following statements is true? Why?
- 0.99999 ...
< 1
- 0.99999 ... = 1
- 0.99999 ...
> 1
Forum thread: Click here. Check out Icarus's (our local mathematician) insightful exposition on this problem.
|
| COLORED DISK SPIN SENSORS |
Imagine a disk spinning like a record player turn table. Half of the disk is black and
the other is white. Assume you have an unlimited number of color sensors. How many
sensors would you have to place around the disk to determine the direction the disk is
spinning? Where would they be placed?
|
| GOLD CHAIN |
a man has a gold chain with 7 links. he needs the service of a laborer for 7 days at a fee of one gold link per day. however, each day of work needs to be paid for separately. in other words, the worker must be paid each day after working and if the laborer is ever overpaid he will quit with the extra money. also he will never allow himself to be owed a link. what is the fewest number of cuts to the chain to facilitate this arrangement and how does that guarantee payment?
|
DUMB CS JOKE
M |
Why do computer programmers always get Christmas and Halloween mixed up?
|
| WATER BUCKETS |
Using only a 5-gallon bucket and a 3-gallon bucket, put exactly four gallons of water in the 5-gallon bucket. (Assume you have an infinite supply of water. No measurement markings on the buckets.)
|
| MASTERMIND I |
This is the logic game of Mastermind. If you haven't played it before, here's how it works. There is a board that is sectioned off into many rows, each row having four slots in which pegs can be inserted. There are six different colors of pegs: green, red, yellow, brown, dark-blue, light-blue. There are two players, A and B. First, A makes up some arrangement of four pegs along a row, the colors and ordering of which are his or her choice. Then B spends the rest of the game trying to guess what A's arrangement is. For every guess that B makes, A will respond by putting some black and/or white pegs right next to A's guess; the black and white pegs are interpreted as follows:
- Black keypeg = one of B's pegs is the correct color and in the correct position
- White keypeg = one of the B's pegs is the correct color but in the wrong position
So if B manages to guess all four colors and positions correctly, A will respond with four black keypegs, and the game is over. The goal is to determine A's secret arrangement in the minimum number of guesses. Below, we see a completed game of Mastermind. Apparently the player was able to determine A's arrangement by using only four guesses. What's is A's arrangement?
|
| TWO-CHILD FAMILY I |
In a two-child family, one child is a boy. What is the probability that the other child is a girl?
|
| TWO-CHILD FAMILY II |
In a two-child family, the older child is a boy. What is the probability that the other child is a girl?
|
| 2 = 1 |
"Proof" that 2 = 1:
| a = b |
| a2 = ab |
| a2 - b2 = ab-b2 |
| (a-b)(a+b) = b(a-b) |
| a+b = b |
| b+b = b |
| 2b = b |
| 2 = 1 |
Does this argument make sense?
|
| BRIDGE CROSSING |
Four people, A, B, C, and D, are on one side of a bridge, and they all want to cross the bridge. However, it's late at night, so you can't cross without a flashlight. They only have one flashlight. Also, the bridge is only strong enough to support the weight of two people at once. The four people all walk at different speeds: A takes 1 minute to cross the bridge, B takes 2 minutes, C takes 5 minutes, and D takes 10 minutes. When two people cross together, sharing the flashlight, they walk at the slower person's rate. How quickly can the four cross the bridge?
Note: Supposedly a classic Microsoft question.
|
CYCLOID
M |
If you drew a dot on the edge of a wheel and traced the path of the dot as the wheel rolled one complete revolution along a line, then the path formed would be called a cycloid (shown in red below), combining both forward and circular motion. What is the length of the path formed by one complete revolution? Assume the wheel has a radius of 1.
|
| COCONUT MONKEY |
Ten people land on a deserted island. There they find lots of coconuts and a monkey. During their first day they gather coconuts and put them all in a community pile. After working all day they decide to sleep and divide them into ten equal piles the next morning. That night one castaway wakes up hungry and decides to take his share early. After dividing up the coconuts he finds he is one coconut short of ten equal piles. He also notices the monkey holding one more coconut. So he tries to take the monkey's coconut to have a total evenly divisible by 10. However when he tries to take it the monkey conks him on the head with it and kills him. Later another castaway wakes up hungry and decides to take his share early. On the way to the coconuts he finds the body of the first castaway, which pleases him because he will now be entitled to 1/9 of the total pile. After dividing them up into nine piles he is again one coconut short and tries to take the monkey's coconut. Again, the monkey conks the man on the head and kills him. One by one each of the remaining castaways goes through the same process, until the 10th person to wake up gets the entire pile for himself. What is the smallest number of possible coconuts in the pile, not counting the monkeys?
|
| PRISONER DILEMMA REDUX |
You and your partner in crime are both arrested and questioned separately. You are offered a chance to confess, in which you agree to testify against you partner, in exchange for all charges being dropped against you, unless he testifies against you also. Your lawyer, whom you trust, says that the evidence against both of you, if neither confesses, is scant and you could expect to take a plea and each serve 3 years. If one implicates the other, the other can expect to serve 20 years. If both implicate each other you could each expect to serve 10 years. You assume the probability of your partner confessing is p. Your highest priority is to keep yourself out of the pokey, and your secondary motive is to keep you partner out. Specifically you are indifferent to you serving x years and your partner serving 2x years. At what value of p are you indifferent to confessing and not confessing?
|
| JAPANESE TEMPLE GEOMETRY I |
Find the side length of the internal square and the radii of the internal circles, in terms of a.
Note: This geometry problem comes from the tradition of sangaku. During the period between 1639 and 1854, many Japanese samurai apparently adopted geometry as a serious mental discipline! They would solve many difficult geometric problems, and carefully inscribe the solutions into beautiful wooden tablets, often with color. Then they would hang their solutions under roofs for public viewing, either to show respect for the elegance of math, or perhaps just to show off their intelligence and challenge other practitioners of sangaku -- a Japanese word that literally means mathematical tablet. Sangaku problems are usually just Euclidean geometry, but others seem almost impossible to do without cheating and using higher level math (e.g. calculus, affine transformations). Also notable is a strong emphasis on ellipses and circles, an emphasis not found in Western studies of geometry.
Note 2: For a hardcopy of the above image only, click here and print. Hardcopies are useful for drawing lines and labeling vertices and what not.
Problem source: Fukagawa, H. and D. Pedoe. Japanese Temple Geometry problems. Charles Babbage Research Foundation: Winnipeg, 1989.
|
| JAPANESE TEMPLE GEOMETRY II |
Find the radii of the internal circles, in terms of a.
Note: For a hardcopy of the above image only, click here and print. Hardcopies are useful for drawing lines and labeling vertices and what not.
Note 2: Thanks a lot to Jasvir Nagra for sending me such a clear solution, even with diagrams drawn with Postscript!
Problem source: Fukagawa, H. and D. Pedoe. Japanese Temple Geometry problems. Charles Babbage Research Foundation: Winnipeg, 1989.
|
| JAPANESE TEMPLE GEOMETRY III |
Find the radii of the internal circles, in terms of a.
Note: For a hardcopy of the above image only, click here and print. Hardcopies are useful for drawing lines and labeling vertices and what not.
Problem source: Fukagawa, H. and D. Pedoe. Japanese Temple Geometry problems. Charles Babbage Research Foundation: Winnipeg, 1989.
|
| MASTERMIND II |
This is the logic game of Mastermind. If you haven't played it before, here's how it works. There is a board that is sectioned off into many rows, each row having four slots in which pegs can be inserted. There are six different colors of pegs: green, red, yellow, brown, dark-blue, light-blue. There are two players, A and B. First, A makes up some arrangement of four pegs along a row, the colors and ordering of which are his or her choice. Then B spends the rest of the game trying to guess what A's arrangement is. For every guess that B makes, A will respond by putting some black and/or white pegs right next to A's guess; the black and white pegs are interpreted as follows:
- Black keypeg = one of B's pegs is the correct color and in the correct position
- White keypeg = one of the B's pegs is the correct color but in the wrong position
So if B manages to guess all four colors and positions correctly, A will respond with four black keypegs, and the game is over. The goal is to determine A's secret arrangement in the minimum number of guesses. Below, we see a completed game of Mastermind. Apparently the player was able to determine A's arrangement by using only four guesses. What's is A's arrangement?
|
| PUNCTUATION II |
Add punctuation to the following phrase to make something gramatically and logically coherent:
i had had had tom had had had had had had had had the praise of the teacher
|
| BROKEN CLOCK |
My fancy new digital alarm clock is broken! The time 'jumps' around.
When I reset it, it reads 12:00:00. Then it runs as it should, but after 12:04:15 it resets back to 12:00:00. It counts up to 12:04:15 again and then it jumps to ... 12:08:32 ! Weird stuff. Do you know what's wrong with my alarm clock?
Note: The contributor, Remco Hartog, actually constructed this riddle himself!
|
| WHAT HAPPENED I |
There's a man lying dead in a telephone booth beside a river. The phone is off the hook, and there is smashed glass on the floor of the phone booth. What happened?
Note: I've always felt uncomfortable with these "what happened" riddles, because it seems like you could design a wide variety fantastic explanations. Indeed, the official answer I've been e-mailed for this riddle is quite fantastic.
|
| BUS PASSENGERS |
The UC Berkeley bus had a minimal number of passengers. When it arrived at Telegraph Avenue, 3/4 of the passengers got out, and 7 people got on. At the next two stops, Shattuck and Hearst, the same thing happened. How many got off at Hearst?
|
| SPRINKLER AND PUMP |
Take one of those spinning sprinklers everyone has seen before, the kind that has two pipes sticking out with the ends slightly bent to the side in opposite directions. Let's say the sprinkler normally turns clockwise when shooting water. Hook a hose from the sprinkler to a water pump. Submerge the sprinkler in water, so that now when you turn on the pump, water is drawn through the sprinkler's pipes. What happens to the sprinkler?
Note: From Surely You're Joking, Mr. Feynman! by Richard Feynman. Originally a problem hotly debated among Princeton physicists in the 1940s. Feynman resolved the problem by conducting an experiment that resulted in the shattering of a glass tank and ultimately getting banned from a lab.
Forum thread: click here
|
| SOLDER CUBE |
Say you have some bendable wires (any number, any length). What is the minimum number of solder connections needed to make a cube? Prove it. Also, what is the minimum number of wires necessary? Prove it.
|
| ANALOG CLOCK III |
Consider an analog clock face on which the hour and minute hands
move smoothly. If we swap the position of the hour and minute
hands, we usually end up with an invalid clock face which never
occurs during the day (the hour hand pointing directly at 12 and
the minute hand at 3, for instance). But there are some times when
these switched hands *do* tell a valid time: specifically, when the
two hands are pointing in the same direction. Are there any other
such times?
Note: From John Leen, one of my CS170 TAs from Spring 2002 at UC Berkeley. An excellent teacher.
|
| PARTY HANDSHAKES |
The master of a college and his wife has decided to throw a party and invited N guest and their spouses. On the night of the party, all guests turned up with their spouse, and they all had a great time. When the party was concluding, the master requested all his guests (including his wife, but not himself) to write down the number of persons they shook hands with, and to put the numbers in a box. When the box was opened, he was surprised to find all integers from 0 to 2N inclusive.
Assuming that a person never shake hands with their own spouse and that no one lied, how many hands did the master shake?
|
| RANDOMIZED ICE CREAM |
A vendor is handing out free ice cream cones in alphabetical order of flavor, each cone being a different flavor. Kids are lined up at the ice cream truck, and you're first in line! The vendor will hand you ice cream cones one at a time, and you must decide whether to keep the cone or pass it on to the next kid in line. The first cone is guaranteed to be chocolate.
You like all flavors equally, so you want to randomly select a cone with each flavor having an equal chance of being chosen. Unfortunately, you don't know the total number of flavors, but being the little hipster that you are, you are carrying a pocket calculator which can generate random numbers from 1 to X, where X is a value you punch in. How can you decide which flavor to keep?
Note: Problem made by Yosen Lin and William Wu! © 2002. Special thanks to the forum regulars for proofreading it.
|
| ANSWER MACHINE HACKING |
Consider an answering machine with remote inquiry facility, where you can call the answering machine and enter a 4 digit passcode into your telephone keypad, so you can listen to the messages from anywhere you like. Many of these machines will let you in if you enter the correct consecutive sequence of digits, regardless of what preceded that sequence.
|
Example: Passcode is 1234.
if you feed the machine 1234, you're in
if you feed the machine 01234, you're in
if you feed the machine 0121234, you're in
if you feed the machine 94129838701234, you're in
|
To brute-force hack the machine, you could try all numbers from 0000 to 9999, sending 40000 sounds across the wire. But since you are a smart hacker, you see that there's room for optimization. What is the shortest series of digits you have to send to the answering machine in order to break the code in any case?
|
| LADDER RUNGS |
You are presented with a ladder. At each stage, you may choose to advance either one rung or two rungs. How many different paths are there to climb to any particular rung; i.e. how many unique ways can you climb to rung "n"?
After you've solved that, generalize. At each stage, you can advance any number of rungs from 1 to K. How many ways are there to climb to rung "n"?
Note: the non-generalized question was asked at a M$ interview.
|
| RACETRACK LAPS |
You are at a track day at your local racecourse in your new Porsche. Because it's a crowded day at the track, you are only allowed to do two laps. You haven't driven your car at the track yet, so you took the first lap easy, at 30 miles per hour. But you do want to see what your ridiculous sports car can do. How fast do you have to go on the second lap to end the day with an average speed of 60 miles per hour?
|
| IMPATIENT PATIENTS |
All patients at the d'Oralian National Hospital require constant care. At least one nurse must be beside a patient's bed at any time and a nurse can only care for one patient simultaniously.
The nurses won't work more that eight hours within any 24 hour period, but don't care when their shift starts. Their shifts look like this: work five hours, one hour break, work another three hours.
How many nurses do you need for one patient? How many nurses do you need for n patients?
Note: Designed by the contributor, Remco Hartog!
|
| E VS PI |
Without computing their actual values, which is greater, e^(pi) or (pi)^e?
|
| COIN FLIP GAME WORTH II |
Game: You continue flipping a coin until you get a tails. I then award you prize money equal to $2^(number of heads).
How much are you willing to pay me to play this game?
|
| COIN FLIP GAME WORTH III |
Game: You continue flipping a coin until the number of heads equals the number of tails. I then award you prize money equal to the number of flips you conducted.
How much are you willing to pay me to play this game?
|
| 271 |
Write 271 as the sum of positive real numbers so as to maximize their product.
|
| LADDER INTO TOWER |
Given a cylindrical tower with a diameter of D and a door of height H from the ground, what is the longest ladder of length L that you could move into the tower and completely enclose? Ignore the thickness of the ladder.
Note: While the contributor was serving in the German military, a superior rank tried to outsmart him with this riddle. It didn't work :)
|
| 6 PERSON ACQUAINTANCE |
Prove that in any group of 6 people, at least 3 must be either mutually acquainted with each other, or mutually unacquainted with each other.
|
| XX |
If x is a positive rational number, prove that x^x is irrational unless x is an integer.
|
| WILLYWUTANG HAS STDS? |
While willywutang was trying to be clever by using only two condoms to satisfy three women, increased friction between overlapped latex layers produces a tear and a dangerous accident! He then becomes very worried about having contracted an STD, especially since one of the women was really skanky looking. Although a random person has only probability 0.001 of having an STD, poor willywutang just can't sleep over those odds. Frantically he hustles to the nearest drugstore, to purchase the ACME All-Purpose STD Checker. The packaging boasts a 0.93 correctness probability. That is, if the user has an STD, the ACME STD Checker will return positive 93% of the time; if the user does not have an STD, it will return negative 93% of the time. Willywutang returns home and uses the checker in his bathroom.
To his dismay, the results are positive.
Assuming that willywutang's promiscuity on average is identical to that of a randomly chosen person, what is the probability that willywutang has STDs?
|
| KABOUTERS |
Kabouters: very small (up to 10 cm) people living in the forests, in mushrooms and roots. Wearing old-fashioned clothes AND they all wear a pointed hat.
Those are kabouters...
In the forests of the Netherlands live a large number of kabouters wearing two different colors of pointy hats. Kabouters consider it very rude to talk about the color of the hat they are wearing, so much so that they don't even know the color of their own hat. They are able to look and distinguish the color of the hats on other kabouters. (They just won't talk about it.) Now every year all kabouters need to be counted and traditionally they present themselves in two groups divided by the color of their hats. How do they do this without talking or communicating the color of their hats to any of the other kabouters?
|
| CIRCUIT FAILURE ANALYSIS |
Consider the circuit shown below. If all components along a path from In to Out are working, then the whole system is considered to be working.
Through appropriate experimentation and modelling, it has been determined that at time T, component Ck fails with probability pk. Also assume that component failure are independent.
What is the probability the system has failed at time T?
Your maintenance technician reports that at time T, component Ck has failed, but she hasn't been able to check any other components yet. What is the probability the system is working? Repeat for k = 1, 2, 3, 4.
|
| RUMOR SPREADING |
You are part of a group of 10 people. All 10 people are in their respective and SEPERATE houses, waiting by the phone. You have a rumor. You pick up the phone to start spreading it and you call someone, beginning a chain such that the nth person to be called will then call and tell it to one other person, except that he will not call himself nor would he (naturally) call the person who just told him (assume, for simplicity, that he WOULD call someone who has told him the rumor before, as long as it was not the person who just called him).
So you make the first call. What is the probability that the 5th call is to you?
Now, can you rework the problem such that a person will NEVER call anyone who has ever told him the rumor before (more realistic, figuring they already know the rumor so why call)? Finally, can you generalize the formula to calculate the probability that the n-th call is to you?
|
| TREES FOR WILLYWUTANG |
So, Willywutang has (somehow) managed to get himself a nice big mansion. The mansion has a nice huge yard in front. However, the yard is completely flat and boring, so Willy decides it'd look nice with a few trees in front. So, he has a landscaper come in to put in some trees. Being the puzzlemeister that he is, Willy decides to give the landscaper a riddle: Plant 9 trees in the yard, so that there are 10 rows of three trees each. Help the poor landscaper decide how to place the trees.
|
| FORCEFIELD DETAINMENT |
A group of prisoners are trapped in a forcefield. These prisoners are perfectly brave, meaning that they would attempt an escape on any positive probability of success. The prisoners are monitored by a guard who has only one bullet in his gun, but who also has perfect marksmanship skills (he never misses). A maintenance technician needs to tune up the forcefield generator, and so for one second, the forcefield is released. How can the guard still keep all the prisoners detained?
|
| WHAT HAPPENED II |
A black man, dressed in black, is crossing a road. He's blind and deaf. A
truck is speeding towards him, with its lights turned off. The street lamps
are also off and there's no moonlight. When the truck is about to hit the man,
the driver hits the brakes and manages to stop just a few centimetres from
him. How did the driver see the man?
|
| WHAT HAPPENED III |
A man walks into a bar and says "I want a coffee and a glass of water. But
make sure the coffee is boiling hot and the water is ice-cold." The barman
says "Sure thing, mr. fireman." The barman had never met him before. How did
he know the man was a fireman?
|
| ONE REAL SOLUTION |
Mathematics departments at some south-western universities received Mr. H.N.ís sly letters asking for the one real solution x of the following two equations:
18 = ((1 + x)18/x)
17 = ((1 + x)17/x)
Professor A.S. at one of those departments sent Mr. H.N. the following brief solution:
18/17 = ((1 + x)18/x) / ((1 + x)17/x) = 1 + x , so x = 1/17
Are there any other real solutions x? Why?
|
| PROOF THAT Y=0 |
Here is a "proof" that y = 0:
Define f(x,y) := (x+y)^2. Now substitute x = u-v, and y=u+v. Taking partial derivatives, we see that
Now the Chain Rule implies
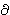 f/ f/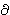 v = ( v = (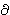 f/ f/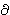 x)( x)(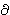 x/ x/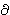 v) + ( v) + (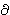 f/ f/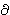 y)( y)(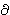 y/ y/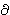 v) = 2(x+y)(-1) + 2(x+y)(1) = 0 v) = 2(x+y)(-1) + 2(x+y)(1) = 0
But the definition of f(u, v) = (u + v)^2 implies also that 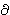 f/ f/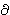 v = 2(u+v) = 2y. Therefore y = 0. Thus we should never divide by variables named "y" while manipulating equations, because y = 0. v = 2(u+v) = 2y. Therefore y = 0. Thus we should never divide by variables named "y" while manipulating equations, because y = 0.
|
COINS SAME WEIGHT
M |
Suppose an odd number (at least three) of coins have the property that, if any one coin is
removed, the rest can be partitioned into two groups each with the same number of coins and also
the same total weight. Show that all the coins must have the same weight.
Note: Linear algebra can be useful here, but I don't know if it's absolutely necessary.
|
| PAPER FOLD RATIO |
A rectangular sheet of paper is folded so that two diagonally opposite corners come together. The crease thus formed is as long as the longer side of the rectangle. What is the ratio of the longer side of the rectangle to the shorter?
|
| FARMER'S ENCLOSURE |
A farmer has four straight pieces of fencing: 1, 2, 3, and 4 yards in length. What is the maximum area he can enclose by connecting the pieces?
|
| GOOD AND BAD COMPUTERS |
You have N computers on a space station. An accident happens, and some of the computers are damaged, but you know the number of good (undamaged) computers is greater than the number of bad (damaged) ones. Your goal is to find *one* computer that's still good.
Your only method of testing is the following: Use one computer (say, X) to test another (Y). If X is a good computer, it tells you correctly the status of Y. If X is bad, it may or may not give the correct status of Y; assume it will give whatever answer is least useful to your testing strategy.
In worst-case, how many tests must you use to find one computer that's still good? (in terms of N)
You're permitted any combination of tests, though keep in mind the bad machines may not be consistent in the results they give you.
|
CHESS STUDY I
C |
White to move. What is optimal play for White?
Note: A very famous study by Richard Reti, dating all the way back to 1921. Reti was a professional in both mathematics and chess; he found the latter more interesting because he could force people to believe the truth of his ideas. He became quite famous in 1924 for being the first to defeat Capablanca in a decade.
|
CHESS STUDY II
C |
White to move. What is optimal play for White?
Note: Another Richard Reti classic study involving the endgame and the surprising power of Kings.
|
| 15-14 SLIDE PUZZLES |
Many of us have had experience with slide puzzles, in which there are tiles numbered from 1 through 15, and you have to slide the tiles such that the numbers are in increasing sequence when read from left to right, top row to bottom. Other variants have fragments of a picture printed on the tiles.
Can you solve the following slide puzzles? If so, list a sequence of moves that produce a solution; if not, explain rigorously. We want the first puzzle to read "1 2 3 4 5 6 7 8 9 10 11 12 13 14 15", and we want the second puzzle to read "Rate Your Mind Pal."
|
| BIRTHDAY PARADOX |
There are N people in one room. How big does N have to be until the probability that at least two people in the room have the same birthday is greater than 50 percent? (Same birthday means same month and day, but not necessarily same year.)
Note: This problem is not really a paradox, but it is given this label because the answer is hard to believe.
|
| WORLD SERIES BETTING |
You dare not offend nor disappoint Great Uncle Willy lest he disown you. He has placed
$2000 in your hands to bet on the outcome of the world series, which is a baseball contest won
by whichever of two teams first wins 4 games. Willyís $2000 was given to you in advance of
the series. At the end of the series he expects you to return to him either $4000 if the team he
chose wins, or nothing if it loses.
But you can find nobody who will accept bets on the entire world series. However, there are
wagerers who will take on even-odds bets, in any amount(s), on each game individually. What
strategy for placing bets on individual games will achieve the cumulative result Willy expects?
Note: This task was reputedly set before job-seekers at a Wall Street brokerage in 1994.
|
| SQUARES ON A GRID |
You're playing a game with your friend on an infinitely large piece of graph paper. You and a friend each have a different color pen. You take turns marking intersections of the grid. The winner is the first person to color 4 intersections that form a square. The square may be of any size, but it must be oriented in the direction of the grid; that is, something like the midpoints of each side of a 2x2 square won't qualify.
What is the optimal strategy for each player?
|
| OBTUSE TRIANGLES |
An obtuse triangle has one of its angles greater than 90 degrees. An acute triangle has all of its angles less than 90 degrees. (A right triangle is neither acute nor obtuse.)
Either find a way to cut up any obtuse triangle into pieces, each of which is an acute triangle, or prove that it can't be done.
Note: "This one is from an old Martin Gardner book. Gardner claims professional mathematicians have been known to get it wrong. Then again, professional mathematicians get the Monty Hall problem wrong sometimes too." - Tim Mann
|
| LOADED CRAPS DICE |
Craps is a 1-player dice game that is played as follows: Roll two 6-sided dice; their sum becomes your "initial" roll. If this initial roll is 2, 3, or 12, you lose. If the initial roll is 7 or 11, you win. Otherwise, keep rolling the dice until you reroll you initial number (and win) or until you roll a 7 (and lose).
You're betting that your adversary is going to lose his game of craps, which should be a favorable bet for you. But you receive an anonymous tip that he's secretly loaded one of the dice, so that it will always come up 5. This increases his chances of winning to 2/3.
Having learned of his evil deed, you're going to secretly load his other die so as to minimize his chance of winning. With what probability should you load each of the six faces? And how does that change his probability of winning?
Note: Writing credits to Matt Lahut.
|
| PENNY TRACK GAME |
There are three one-dimensional tracks, of length 12, 7, and 5 spaces respectively. You start with pennies in the first space of each track; your opponent starts with pennies in the last space of each track. On your turn, you may move any one of your pennies any number of spaces in either direction along a track (as a chess rook), however you are not permitted to bypass the other player's penny or occupy its space. If a player has no legal move, he loses.
What should your first move be?
Note: Hardcore puzzlers will probably solve this immediately, but newer people might take quite some time. For the newer people, the solution to this is a useful trick to have in your random-puzzle-solving arsenal. As a sub-problem, you may want to try solving it without the 5-length track.
|
| POSITIVE MATRIX SUMS |
You have an m x n grid with some real numbers in each cell. You can multiply any row by -1, or any column by -1. Show that by making multiplications of this kind, the sum of the numbers in every row, and the sum of the numbers in every column, can all be made non-negative simultaneously.
|
| TETRAMINOES ON A CHECKERBOARD |
Shown to the below-left is a checkerboard mutilated by the removal of two squares from each of two opposite corners. Shown to the below-right are two T-shaped tiles, each of which can cover four squares of the checkerboard exactly.
If tiles of both kinds are abundant, and if tiles may be rotated, can the mutilated checkerboard be covered exactly with nonoverlapping tiles that match the colors of covered squares? Why?
|
| ALWAYS AHEAD |
In an presidential election between Willywutang and Billybutane, the winning candidate Willywutang has received n+k votes, whereas Billybutane has received n votes. (n and k are positive integers.) If ballots are counted in a random order, what is the probability that Willywutang's accumulating count will always lead his opponent's, and why?
Note: Cute eh? Also a practical calculation ... well, maybe.
|
| CALLING A CARD'S COLOR |
A deck of 26 red and 26 black cards is shuffled into random order and placed face down. Then
the cards are turned up one by one and observed by a guesser. He gets one guess: At a moment of
his choice he may assert that the next card will turn up red. After this card is turned up the game
ends and he wins if his assertion was correct, loses otherwise. And if he doesn't guess at all by the time all cards have been dealt, he loses by default. What guessing policy chosen in
advance maximizes his chance of winning?
|
| COMPLEX MULTIPLICATION |
Consider computing the product of two complex numbers (a + bi) and (c + di). By foiling the polynomials as we learned in grade school, we get
a + bi
c + di
----------
adi - bd
ca + cbi
----------------
(ca - bd) + (ad + cb)i
Note that this standard method uses 4 multiplications and 2 additions to compute the product. (The plus sign in between (ca - bd) and (ad + cb)i does not count as an addition. Think of a complex number as simply a 2-tuple.)
It is actually possible to compute this complex product using only 3 multiplications and 3 additions. From a logic design perspective, this is preferable since multiplications are more expensive to implement than additions. Can you figure out how to do this?
|
| CRAZY SPRINKLER |
A little while ago, Willy Wutang was thinking about the Sprinkler and Pump puzzle. Willy, having a creative mind (or at least a well-developed sense of mischief) had a flash of inspiration, and came up with a very novel sprinkler design. However, before he can patent his new sprinkler, Willy must figure out what it does--his lawyer says it's a necessary part of the patent process.
-
First of all, does the sprinkler turn? If so, in which direction does it turn?
-
Second, what do the jets of water do? In the picture above, the sprinkler is shown at the instant just before the jets of water collide. However, in the picture the sprinkler is being held still, so that even if it wants to turn, it cannot.
-
Third, does the behaviour of the jets of water depend on whether or not the sprinkler is allowed to rotate? Does it maybe depend on how fast the sprinkler rotates?
Note: Writing credits to James Fingas!
|
| CONWAY SEQUENCE |
What row of numbers comes next?
1
11
21
1211
111221
312211
13112221
Note: Mathematician John Conway spent considerable time studying this sequence.
|
| CRYPTIC ADDRESS |
Willy Wutang is writing to his overseas sweetheart. He writes a steamy love letter, seals the envelope, and then inscribes the following cryptic address on the front:
Wood, S
April
England
Strangely enough, the letter reaches its intended recipient. What is Willy's sweetheart's address?
Note: Writing credits to James Fingas :)
|
| FINGER GAUGES |
Willy Wutang is making a robot, and he has bought himself a set of 24 finger gauges. For those of you who have never seen a set of these, each gauge is a strip of metal about 1/2" wide and about one foot long. Each is a different thickness (0.001" up to 0.024"). Each has a hole in one end, and they are fastened together with a nut and bolt, in order of thickness.
Willy's calculations indicate that, with the flame thrower attachment extended, he must adjust the spark plug to be 0.086" from the jellied gasoline nozzle. He pulls out his trusty finger gauge set, and realizes they only go up to 0.024". How can he accurately set the spark plug distance?
If you've figured out how Willy measured 0.086", what is the smallest measurement (in thousandths of an inch) that Willy can't make using his set of feeler gauges? What is the smallest measurement (in thousandths of an inch) that Willy can't approximate to +/-0.001"?
Note: Writing credits to the prolific James Fingas.
|
| ACROBAT THIEF AND GOLD ROPES |
An acrobat thief enters an ancient temple, and finds the following scenario:
- The roof of the temple is 100 meters high.
- In the roof there are two holes, separated by 1 meter.
- Through each hole passes a single gold rope, each going all the way to the floor.
- There is nothing else in the room.
The thief would like to cut and steal as much of the ropes as he can. However, he knows that if he falls from height that is greater than 10 meters, he will die. The only thing in his possession is a knife.
How much length of rope can the acrobat thief get? And how?
|
| SUM OF RECIPROCALS |
Show that the sum of the reciprocals of the first n positive numbers is not an integer for n > 1.
|
| CAKE DIVISION |
Willywutang is holding a birthday party. Either p or q people are going to show up, where p and q are relatively prime numbers. Willy wants to cut the cake beforehand, so his guests will not waste time waiting for slices to be carved out. What is the smallest number of slices Willy must cut out such that every guest can be given the same amount of cake, regardless of whether p or q people show up? The slices do not have to be equally sized, and each guest could receive more than one slice. Also, Willy is not allowed to eat the whole cake and give nothing to all the guests. Also, the entire cake must be distributed amongst the guests -- there can be no leftovers.
Note 1: Two numbers are relatively prime if they share no common factors. So for example, the numbers 4 and 9 are relatively prime, but the numbers 10 and 15 are not because they share the number 5 as a common factor.
Note 2: (5/27/2003 2:17PM) Edited to specify that the entire cake must be given away.
|
| LEMMING DROWNINGS |
1/27/2003 8:30PM
Somewhere in Northern Eurasia, a group of 20 lemmings is planning a special group suicide this year. Each of the lemmings will be placed in a random position along a thin, 100 meter long plank of wood which is floating in the sea. Each lemming is equally likely to be facing either end of the plank. At time t=0, all the lemmings walk forward at a slow speed of 1 meter per minute. If a lemming bumps into another lemming, the two both reverse directions. If a lemming falls off the plank, he drowns. What is the longest time that must elapse till all the lemmings have drowned?
Author: William Wu
Note 1: If you make a certain observation, the calculations required become very trivial!
Note 2: Yes, I know Lemmings don't really commit group suicides. Problem and storyline was inspired by the Lemmings video game.
|
| RESISTOR CUBE |
1/27/2003 8:30PM
Imagine a cube where each edge is a 1 ohm resistor. Find the resistance between opposite corners of the cube.
There are many ways to solve this problem, but some ways are more clever than others.
|
ASTROWRENCH
P |
1/27/2003 8:30PM
Two astronauts are standing on a spinning space station shaped like a disk. They are the same radial distance away from the disk's center, and standing opposite to each other across from the center (e.g., if you draw a line connecting the two astronauts, the line crosses the disk's center.) One astronaut wants to toss a wrench to the other. Among the infinitude of trajectories which will accomplish this goal, characterize one of the trajectories without writing a single equation.
Note: Be wary grasshopper. There are several common wrong answers to this problem!
|
| SLASH AND DASH SEQUENCE |
2/2/2003 3:31AM
What are the next few terms in the sequence?
-
/-\
/-\-
//-\\
/-\--
/-\/-\
/-\---
///-\\\
//-\\-
/-\-/-\
Hint 1: Maybe it'll go easier if you replace - with 0, and the / and \ with ( and ). Or not.
Hint 2: These are just the numbers 1 through 10, expressed differently. And despite the presence of zero, you can't express zero this way.
Hint 3: All your base are belong to prime numbers.
|
| JEWEL THIEVES |
2/2/2003 3:31AM
Two thieves conspire to steal a valuable necklace made of diamonds and rubies (evenly spaced, but not necessarily alternating or symmetric). After they take it home, they decide that the only way to divide the booty fairly is to physically cut the necklace in half.
Prove that, if there is an even number of diamonds and an even number of rubies, it's possible to cut the necklace into two pieces, each of which contains half the diamonds and half the rubies.
|
| WIRECUFFS |
3/9/2003 4:11AM
In a cliche effort to illustrate the importance of teamwork-oriented problem solving, the Boss has chained Dilbert to Carol The Secretary via wire wrapped around their wrists, as shown in the following snapshot:
The goal is for Dilbert and Carol to unlink themselves from each other; considering what a horrible woman Carol is, Dilbert wouldn't have it any other way. The wire is unbreakable, and as much as Dilbert would like to saw off Carol's limbs, that's against company policy. How can Dilbert and Carol get away from each other?
|
BUFFON'S NEEDLE
M |
4/7/2003 12:47AM
While recovering from wounds in the American Civil War, a Captain Fox threw needles at a surface ruled with parallel lines, and was thus able to experimentally infer the value of pi. True story! To find out exactly how pi plays into this scenario, solve the following problem: A surface is ruled with parallel lines. The lines are at distance D apart from each other. Suppose we throw a needle of length L on the surface at random. What is the probability that the needle will intersect one of the lines?
Note 1: A famous problem posed and solved in 1777 by French naturalist Buffon. It has long since fascinated scientists, and marks the origin of geometrical probability -- the analysis of geometrical configurations of randomly placed objects.
Note 2: These 19th century experiments began development of the Monte Carlo method, which uses repeated simulation to approximate true statistics.
Note 3: This isn't much of a riddle ... more like just an interesting mathematical exercise. The most challenging part is setting up the problem.
|
| SAMWISE AND GANDALF |
4/7/2003 12:47AM
Samwise Gamgee has a square plot of land, each side being 1 unit. One day, Sam finds out that the dark Lord Sauron has a telephone line that he uses to speak with a traitor amongst the hobbits.
Gandalf informs him that the telephone line runs in a straight line parallel to the ground and passes beneath the square plot of land, but he does not know its location. Sam decides to dig up around the perimeter of his land to discover the telephone line, but Gandalf says it is not necessary to dig around the entire length of 4 units.
Sam brightens up, and says "I know what you mean. I can just dig 3 sides and still discover it. For even if the phone line runs along the fourth side, I will still detect it at the end points ! "
Gandalf shakes his head. "No, Sam. You are on the right track, but you can do better than that."
What solution does Gandalf have in mind for the optimum length of the "digging curve" ?
|
RETROGRADE CHESS I
C |
4/7/2003 12:47AM
What was the last move made?
Note: From Karl Fabel's 1955 book Rund um das Schachbrett.
|
| PATH COLLISION |
5/11/2003 10:44PM
Consider an N x N grid. Denote one corner as point A, and the opposite corner as point B. George is walking from A to B, and Lennie is walking from B to A. All paths are equally likely, as long as they follow the grid and never move away from the destination. (Hence George's path can never move down or left, and Lennie's path can never move up or right.)
- What is the probability that George and Lennie collide?
- If George runs and thus moves three times faster than Lennie, what is the probability of collision?
|
| ROPE AROUND THE EARTH |
5/11/2003 10:44PM
Assume the Earth is a perfect sphere of radius r and suppose a rope of zero elasticity is tied tightly around it.
One metre is now added to the rope's length. If the rope is now pulled at one point as high as possible above the Earth's surface, what height will be reached?
|
| MOLINA'S URNS |
5/11/2003 10:44PM
Two urns contain the same total numbers of balls, some blacks and some whites in each. From each urn are drawn n balls with replacement, where n >= 3. Find the number of drawings and the composition of the two urns so that the probability that all white balls are drawn from the first urn is equal to the probability that the drawing from the second is either all whites or all blacks.
Author: E.C. Molina.
|
| TWO GAMBLING MACHINES |
5/27/2003 2:24PM
Consider a gambling machine A. When you put in $X and pull the handle, it will spit out (equally likely) either $0.7*X, $0.8*X, $0.9*X, $1.1*X, $1.2*X, or $1.5*X.
Now consider the following two ways of playing this machine:
- Put in $1, pull the handle, and keep whatever you get. Repeat.
- Initially, put in $1. Pull the handle, then put in whatever you get. Repeat.
Can you win money with this machine? Which is the better way to play? How can this be?
|
| HEXAGON IN CIRCLE |
5/11/2003 10:44PM
A hexagon with sides of length 2, 7, 2, 11, 7, 11 is inscribed in a circle. Find the radius of the circle.
|
| ELEGANTLY GREEDY PIRATES |
Contributor: Mark Newheiser
|
5:15 PM 8/3/2004
|
You have 1000 pirates, who are all extremely greedy, heartless, and
perfectly rational. They're also aware that all the other pirates
share these characteristics. They're all ranked by the order in which
they joined the group, from pirate one down to a thousand.
They've stumbled across a huge horde of treasure, and they have to
decide how to split it up. Every day they will vote to either kill the
lowest ranking pirate, or split the treasure up among the surviving
pirates. If 50% or more of them vote to split it, the treasure gets
split. Otherwise, they kill the lowest ranking pirate and repeat the
process until half or more of the pirates decide to split the
treasure.
The question, of course, is at what point will the treasure be split,
and what will the precise vote be?
After that, consider solving the problem when a two-thirds or three-fourths majority is required. Try to generalize the result.
|
| FAIR COIN GAMBLING |
Contributor: William Wu
|
9:00 PM 8/19/2004
|
Starting with 0 dollars, you repeatedly flip a fair coin, earning $1 each time heads appears, and losing $1 each time tails appears. When your net cash reaches either A or -B, you stop gambling.
- As a function of A and B, compute the expected number of flips until the game stops.
- Now consider the unstopped version of this process, in which you gamble indefinitely regardless of your current profit or debt. Prove that the expected time till your net cash is +$1 is infinite. Likewise, the expected time till your net cash is -$1 is also infinite. And yet one of these two events must occur upon the first coin flip!
Forum threads: More Heads Than Tails and Lazy hunter catch the rabbit.
|
| JUMPING FROG ON LADDER |
Contributor: William Wu
|
9:00 PM 8/19/2004
|
Imagine a upright ladder with n rungs. At each time step, a frog jumps to one of the n rungs uniformly at random. (The frog could jump in place.) Assume time starts counting from 1, and the initial rung position is also chosen uniformly at random.
As the number of rungs tends to infinity, what is the expected time till the frog first jumps downwards? Prove it.
Author: William Wu
|
| COLORING THE RATIONAL PLANE |
Contributor: Eigenray
|
9:00 PM 8/19/2004
|
Let Q denote the set of rationals. How many colors are required to color every point of Q^k in such a way that no two points a unit distance apart are the same color?
- Easy: k = 1
- Medium: k=2,3
- Hard: k>3
|
| RANDOM POINTS ON SPHERE |
Contributor: Joc Koelman
|
9:00 PM 8/19/2004
|
Two points on the surface of a sphere are drawn uniformly at random. What is the maximum likelihood estimate of the distance between these two points? The answer may be shocking at first. After getting the answer, try to explain it intuitively.
|
| NUMBER OF MIDPOINTS |
Contributor: Aryabhatta
|
9:00 PM 8/19/2004
|
You are given n>=2 points in the 2-D plane. For each pair of points (P,Q) from the n points, mark the midpoint of PQ red. Show that there are at least 2n-3 distinct red points. For each n, show an arrangement of n points for which there are exactly 2n-3 distinct red points.
Source: Iranian Mathematical Olympiad
|