 Author
Author |
Topic: Triangles in Z^n (Read 801 times) |
|
Eigenray
wu::riddles Moderator
Uberpuzzler
    

Gender: 
Posts: 1948
|
 |
Triangles in Z^n
« on: Aug 30th, 2007, 7:14pm » |
 Quote Quote  Modify Modify
|
When can a triangle be embedded (up to similarity) in  n? n?
|
|
 IP Logged IP Logged |
|
|
|
Barukh
Uberpuzzler
    

Gender: 
Posts: 2276
|
 |
Re: Triangles in Z^n
« Reply #1 on: Aug 31st, 2007, 11:27pm » |
 Quote Quote  Modify Modify
|
Let one of the vertices be put at the origin, and two others at lattice points A, B in  n. If a, b are corresponding vectors from the origin to points A, B, then the area S of the triangle satisfies: n. If a, b are corresponding vectors from the origin to points A, B, then the area S of the triangle satisfies:
(2S)2 = a2b2 - (ab)2,
which is an integer. Therefore, if S is not a square root of an integer, the triangle cannot be embedded.
Of course, this is not suficient to answer the original question, even in general.
|
| « Last Edit: Aug 31st, 2007, 11:28pm by Barukh » |
 IP Logged IP Logged |
|
|
|
Barukh
Uberpuzzler
    

Gender: 
Posts: 2276
|
 |
Re: Triangles in Z^n
« Reply #2 on: Sep 1st, 2007, 5:08am » |
 Quote Quote  Modify Modify
|
Hereís another necessary condition: If a triangle with angles 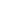 , , 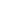 is embeddable in is embeddable in  n, then both quantities tan2( n, then both quantities tan2(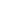 ) and tan( ) and tan(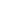 )/tan( )/tan(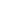 ) are rational. ) are rational.
This condition, I think, is also sufficient.

|
| « Last Edit: Sep 1st, 2007, 5:13am by Barukh » |
 IP Logged IP Logged |
|
|
|
Michael Dagg
Senior Riddler
   

Gender: 
Posts: 500
|
 |
Re: Triangles in Z^n
« Reply #3 on: Sep 1st, 2007, 7:59am » |
 Quote Quote  Modify Modify
|
Good question. Obviously the squares of the ratios of the side lengths
will have to be rational. But that's not enough: a classic question asks,
"Is there an equilateral triangle in † Z^n † ?" ; here the ratios of the
side lengths are all equal to †1.
But, there are no equilateral triangles in † Z^n †by Pick's Theorem.
|
| « Last Edit: Sep 1st, 2007, 8:05am by Michael Dagg » |
 IP Logged IP Logged |
Regards,
Michael Dagg
|
|
|
Eigenray
wu::riddles Moderator
Uberpuzzler
    

Gender: 
Posts: 1948
|
 |
Re: Triangles in Z^n
« Reply #4 on: Sep 1st, 2007, 10:24am » |
 Quote Quote  Modify Modify
|
on Aug 31st, 2007, 11:27pm, Barukh wrote:| Therefore, if S is not a square root of an integer, the triangle cannot be embedded. |
|
But I am asking about similarity classes of triangles.
on Sep 1st, 2007, 5:08am, Barukh wrote:If a triangle with angles 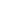 , , 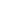 is embeddable in is embeddable in  n, then both quantities tan2( n, then both quantities tan2(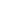 ) and tan( ) and tan(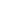 )/tan( )/tan(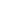 ) are rational. ) are rational.
This condition, I think, is also sufficient. |
|
It is in fact sufficient for the triangle to be embeddable in some  n. n.
But for each n, which triangles can be embedded in  n? It may help to first consider the case n=2. n? It may help to first consider the case n=2.
on Sep 1st, 2007, 7:59am, Michael_Dagg wrote:| But, there are no equilateral triangles in Z^n by Pick's Theorem. |
|
Not in  2, but there are in all higher dimensions. In fact, a regular n-simplex can be embedded in 2, but there are in all higher dimensions. In fact, a regular n-simplex can be embedded in  n when: n when:
n is even and n+1 is a square;
n  3 mod 4; or 3 mod 4; or
n  1 mod 4 and n+1 is the sum of two squares. 1 mod 4 and n+1 is the sum of two squares.
|
| « Last Edit: Sep 1st, 2007, 10:28am by Eigenray » |
 IP Logged IP Logged |
|
|
|
Barukh
Uberpuzzler
    

Gender: 
Posts: 2276
|
 |
Re: Triangles in Z^n
« Reply #5 on: Sep 2nd, 2007, 5:20am » |
 Quote Quote  Modify Modify
|
on Sep 1st, 2007, 10:24am, Eigenray wrote:
It may help to first consider the case n=2. |
|
This case is easy: tan(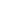 ), tan( ), tan(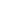 ) both rational. ) both rational.
Proof: Let tan(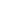 ) = p/q, tan( ) = p/q, tan(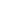 ) = r/s. Then the lattice points (0, rp), (ps, 0), (rq, 0) form a triangle similar to given. ) = r/s. Then the lattice points (0, rp), (ps, 0), (rq, 0) form a triangle similar to given.
The converse may be shown using formulas for tan of the sum of two angles.
|
|
 IP Logged IP Logged |
|
|
|
Michael Dagg
Senior Riddler
   

Gender: 
Posts: 500
|
 |
Re: Triangles in Z^n
« Reply #6 on: Sep 2nd, 2007, 11:21am » |
 Quote Quote  Modify Modify
|
I meant Z^2 when I referred to Pick's Theorem.
I don't think I've ever seen your result about the n-simplex.
I guess I can imagine a proof (assuming "when" means "if", not "iff").
Is there a simple way to handle the case n=2? I mean, besides the
Pick's Theorem method I mentioned, which I've always thought was a
wonderful use of an unexpected tool...
|
|
 IP Logged IP Logged |
Regards,
Michael Dagg
|
|
|
Eigenray
wu::riddles Moderator
Uberpuzzler
    

Gender: 
Posts: 1948
|
 |
Re: Triangles in Z^n
« Reply #7 on: Sep 2nd, 2007, 12:03pm » |
 Quote Quote  Modify Modify
|
on Sep 2nd, 2007, 5:20am, Barukh wrote:
Proof: Let tan(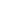 ) = p/q, tan( ) = p/q, tan(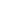 ) = r/s. Then the lattice points (0, rp), (ps, 0), (rq, 0) form a triangle similar to given. ) = r/s. Then the lattice points (0, rp), (ps, 0), (rq, 0) form a triangle similar to given. |
|
Now can you think of a way to generalize that same construction?
on Sep 2nd, 2007, 11:21am, Michael_Dagg wrote:I don't think I've ever seen your result about the n-simplex.
I guess I can imagine a proof (assuming "when" means "if", not "iff").
Is there a simple way to handle the case n=2? I mean, besides the
Pick's Theorem method I mentioned, which I've always thought was a
wonderful use of an unexpected tool... |
|
It is in fact iff, and it's proved in:
I. J. Schoenberg, Regular simplices and quadratic forms, J. London Math. Soc., 12 (1937) 48-55.
The relation to quadratic forms is this:
Lemma: Let  = OP1...Pn and = OP1...Pn and  ' = OP1'...Pn' be two given n-dimensional simplices, and let ' = OP1'...Pn' be two given n-dimensional simplices, and let
F(x) =  i,k <Pi, Pk> xixk, F(y) = i,k <Pi, Pk> xixk, F(y) =  i,k <Pi', Pk'> yiyk i,k <Pi', Pk'> yiyk
be their corresponding quadratic forms. Let L( ) and L( ) and L( ') be the lattices generated by the sets of vectors {Pi} and {Pi'}. A multiple ') be the lattices generated by the sets of vectors {Pi} and {Pi'}. A multiple  L( L( ) can be inscribed in L( ) can be inscribed in L( ') iff the quadratic forms F(x) and F'(y) are rationally equivalent up to a factor. ') iff the quadratic forms F(x) and F'(y) are rationally equivalent up to a factor.
However, the case n even is relatively simple: just consider the volume.
|
|
 IP Logged IP Logged |
|
|
|
Barukh
Uberpuzzler
    

Gender: 
Posts: 2276
|
 |
Re: Triangles in Z^n
« Reply #8 on: Sep 4th, 2007, 10:18am » |
 Quote Quote  Modify Modify
|
on Sep 2nd, 2007, 12:03pm, Eigenray wrote:
Now can you think of a way to generalize that same construction? |
|
In fact, I've read Beeson's paper...
|
|
 IP Logged IP Logged |
|
|
|
Barukh
Uberpuzzler
    

Gender: 
Posts: 2276
|
 |
Re: Triangles in Z^n
« Reply #9 on: Sep 7th, 2007, 10:05am » |
 Quote Quote  Modify Modify
|
Hereís what I know.
The embeddability of a triangle with rational tan2(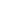 ) = p/q in ) = p/q in  n implies a non-trivial solution of the following system of Diophantine equations (indices run 1 ... n): n implies a non-trivial solution of the following system of Diophantine equations (indices run 1 ... n):
pq  ai2 = ai2 =  hi2, hi2,
 aihi = 0 aihi = 0
where a, h are unknowns.
It then follows that if pq is represented as a sum of n squares, the corresponding angle is embeddable in  n+1. Therefore, any triangle with rational tangent squares may be embedded in n+1. Therefore, any triangle with rational tangent squares may be embedded in  5. 5.
Thatís sufficient but not necessary. Itís further shown that if triangle is embeddable in  4, then itís embeddable also in 4, then itís embeddable also in  3. The trivial example of this is the equilateral triangle: for it, pq = 3, a sum of three squares, and embeddable in three dimensional space. 3. The trivial example of this is the equilateral triangle: for it, pq = 3, a sum of three squares, and embeddable in three dimensional space.
|
| « Last Edit: Sep 7th, 2007, 10:06am by Barukh » |
 IP Logged IP Logged |
|
|
|
|
 WRITE MATH!
WRITE MATH!
 Home
Home  Help
Help  Search
Search  Members
Members  Login
Login  Register
Register WRITE MATH!
WRITE MATH!
 Home
Home  Help
Help  Search
Search  Members
Members  Login
Login  Register
Register